Published online by Cambridge University Press: 24 October 2008
Let p be a prime and let f(x) be a quartic polynomial with integral coefficients. I consider the problem of estimating the least non-negative non-residue k of f(x) (modp) (I omit the mod p hereafter), for large primes p, so f(x) ≡ r has a solution for
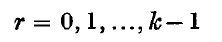
but not for r = k. The same problem for cubics has been considered by Mordell ((1)), who showed that

as p → ∞, where the constant implied in the O-symbol is independent of the coefficients of the cubic. In fact a more detailed examination of Mordell's proof gives the better estimate

It is the purpose of this paper to show that this same estimate also holds for quartic polynomials.