Article contents
On the set of the logarithm of the LMO invariant for integral homology 3-spheres
Published online by Cambridge University Press: 01 September 2008
Abstract
The LMO invariant is a very strong invariant such that it is expected to classify integral homology 3-spheres. In this paper we identify the set of the degree ≤ 6 parts of the logarithm of the LMO invariant for integral homology 3-spheres. As an application, we obtain a complete set of relations which characterize the set of Ohtsuki's invariants {λi(M)} for i ≤ 6. For any simple Lie algebra 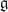 , we also obtain a complete set of relations which characterize the set of perturbative P
, we also obtain a complete set of relations which characterize the set of perturbative P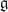 invariants {
invariants {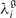 (M)} for i ≤ 3.
(M)} for i ≤ 3.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 145 , Issue 2 , September 2008 , pp. 349 - 361
- Copyright
- Copyright © Cambridge Philosophical Society 2008
References
REFERENCES
- 1
- Cited by


