Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Cowie, E. R.
1983.
A note on uniquely maximal Banach spaces.
Proceedings of the Edinburgh Mathematical Society,
Vol. 26,
Issue. 1,
p.
85.
Rosenthal, Haskell
1985.
Banach Spaces.
Vol. 1166,
Issue. ,
p.
129.
Legǐsa, Peter
1986.
Advances in Invariant Subspaces and Other Results of Operator Theory.
p.
213.
Arazy, Jonathan
1987.
Geometrical Aspects of Functional Analysis.
Vol. 1267,
Issue. ,
p.
122.
Legiša, P.
1989.
Banach spaces with a shrinking hyperorthogonal basis.
Acta Mathematica Hungarica,
Vol. 54,
Issue. 3-4,
p.
181.
Jamison, J. E.
and
Lin, Pei-Kee
1989.
A result on hermitian operators.
Glasgow Mathematical Journal,
Vol. 31,
Issue. 1,
p.
71.
Kamińska, Anna
1994.
Isometries of Musielak-Orlicz Spaces Equipped with the Orlicz Norm.
Rocky Mountain Journal of Mathematics,
Vol. 24,
Issue. 4,
Randrianantoanina, Beata
1998.
Contractive Projections and Isometries in Sequence Spaces.
Rocky Mountain Journal of Mathematics,
Vol. 28,
Issue. 1,
Randrianantoanina, Beata
1998.
One-Complemented Subspaces of Real Sequence Spaces.
Results in Mathematics,
Vol. 33,
Issue. 1-2,
p.
139.
Guerrero, Julio Becerra
and
Palacios, Angel Rodriguez
2000.
Isometric Reflections on Banach Spaces after a Paper of A. Skorik and M. Zaidenberg.
Rocky Mountain Journal of Mathematics,
Vol. 30,
Issue. 1,
Hornor, William
and
Jamison, James E.
2001.
Isometries of some Banach spaces of analytic functions.
Integral Equations and Operator Theory,
Vol. 41,
Issue. 4,
p.
410.
Casazza, Peter G.
2001.
Vol. 1,
Issue. ,
p.
271.
Chalmers, B.L.
and
Ostrovskii, M.I.
2004.
A New Class of Normed Spaces with Nontrivial Groups of Isometries and Some Estimates for Operators with Given Action.
Rocky Mountain Journal of Mathematics,
Vol. 34,
Issue. 1,
Jamison, James E.
2007.
Bicircular projections on some Banach spaces.
Linear Algebra and its Applications,
Vol. 420,
Issue. 1,
p.
29.
Godefroy, G.
2014.
Obituary: Nigel John Kalton, 1946–2010.
Bulletin of the London Mathematical Society,
Vol. 46,
Issue. 6,
p.
1292.
Dilworth, S.J.
and
Randrianantoanina, B.
2015.
On an isomorphic Banach–Mazur rotation problem and maximal norms in Banach spaces.
Journal of Functional Analysis,
Vol. 268,
Issue. 6,
p.
1587.
Ferenczi, Valentin
and
Rosendal, Christian
2017.
NON-UNITARISABLE REPRESENTATIONS AND MAXIMAL SYMMETRY.
Journal of the Institute of Mathematics of Jussieu,
Vol. 16,
Issue. 2,
p.
421.
Moslehian, M. S.
Muñoz-Fernández, G. A.
Peralta, A. M.
and
Seoane-Sepúlveda, J. B.
2022.
Similarities and differences between real and complex Banach spaces: an overview and recent developments.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 116,
Issue. 2,
Cabello Sánchez, Félix
Ferenczi, Valentin
and
Randrianantoanina, Beata
2022.
On Mazur rotations problem and its multidimensional versions.
São Paulo Journal of Mathematical Sciences,
Vol. 16,
Issue. 1,
p.
406.


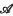 } it vectors such that, for each α, there is an hermitian (in the numerical range sense, see (4)) projection
} it vectors such that, for each α, there is an hermitian (in the numerical range sense, see (4)) projection 