Article contents
Prevalent Lq-dimensions of measures
Published online by Cambridge University Press: 24 June 2010
Abstract
Let K be a compact subset of ℝd and write s for the Hausdorff dimension of K. For a probability measure μ on K, the lower and upper Lq-dimensions of order q ∈ ℝ are defined by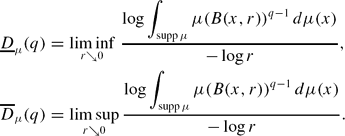 In this paper we study Lq-dimensions of measures that are generic in the sense of prevalence. In particular, we prove that if K satisfies a mild regularity condition, then a prevalent probability measure μ on K satisfies:
In this paper we study Lq-dimensions of measures that are generic in the sense of prevalence. In particular, we prove that if K satisfies a mild regularity condition, then a prevalent probability measure μ on K satisfies: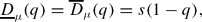 for all 0 ≤ q ≤ 1, and
for all 0 ≤ q ≤ 1, and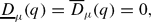 for all 1 ≤ q. This result is in sharp contrast to the behaviour of the Lq-dimensions of measures that are generic in the sense of Baire category. Namely, if K satisfies a mild regularity condition, then a probability measure μ on K that is generic in the sense of Baire category satisfies:
for all 1 ≤ q. This result is in sharp contrast to the behaviour of the Lq-dimensions of measures that are generic in the sense of Baire category. Namely, if K satisfies a mild regularity condition, then a probability measure μ on K that is generic in the sense of Baire category satisfies: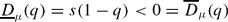 for all 1 < q.
for all 1 < q.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 149 , Issue 3 , November 2010 , pp. 553 - 571
- Copyright
- Copyright © Cambridge Philosophical Society 2010
References
REFERENCES
- 3
- Cited by


