Published online by Cambridge University Press: 01 January 2009
A notion of good behavior is introduced for a definable subcategory 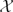 of left R-modules. It is proved that every finitely presented left R-module has a pure projective left
of left R-modules. It is proved that every finitely presented left R-module has a pure projective left 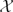 -approximation if and only if the associated torsion class
-approximation if and only if the associated torsion class 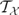 of finite type in the functor category (mod-R, Ab) is coherent, i.e., the torsion subobject of every finitely presented object is finitely presented. This yields a bijective correspondence between such well-behaved definable subcategories and preenveloping subcategories
of finite type in the functor category (mod-R, Ab) is coherent, i.e., the torsion subobject of every finitely presented object is finitely presented. This yields a bijective correspondence between such well-behaved definable subcategories and preenveloping subcategories  of the category Add(R-mod) of pure projective left R-modules. An example is given of a preenveloping subcategory
of the category Add(R-mod) of pure projective left R-modules. An example is given of a preenveloping subcategory  ⊆ Add(R-mod) that does not arise from a covariantly finite subcategory of finitely presented left R-modules. As a general example of this good behavior, it is shown that if R is a ring over which every left cotorsion R-module is pure injective, then the smallest definable subcategory
⊆ Add(R-mod) that does not arise from a covariantly finite subcategory of finitely presented left R-modules. As a general example of this good behavior, it is shown that if R is a ring over which every left cotorsion R-module is pure injective, then the smallest definable subcategory 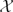 (R-proj) containing every finitely generated projective module is well-behaved.
(R-proj) containing every finitely generated projective module is well-behaved.