No CrossRef data available.
Published online by Cambridge University Press: 24 October 2008
For unexplained terms in semigroup theory see [1] or [4].
Let C, D be classes of semigroups such that every finite semigroup in the class C is embeddable in a finite semigroup in the class D. If n ≥ 2 then k is said to be a C – Dcover of n if every semigroup of order n in the class C is embeddable in a semigroup in the class D of order not greater than k. Let 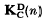 be the least C – D cover of n.
be the least C – D cover of n.