Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Stromberg, Karl
1968.
Large families of singular measures having absolutely continuous convolution squares.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 64,
Issue. 4,
p.
1015.
Taylor, Joseph L.
1969.
𝐿-subalgebras of 𝑀(𝐺).
Transactions of the American Mathematical Society,
Vol. 135,
Issue. 0,
p.
105.
Liu, Teng‐Sun
and
Rooij, Arnoud Van
1969.
Sums and Intersections of Normed Linear Spaces.
Mathematische Nachrichten,
Vol. 42,
Issue. 1-3,
p.
29.
Graham, Colin C.
1971.
𝑀₀(𝐺) is not a prime 𝐿-ideal of measures.
Proceedings of the American Mathematical Society,
Vol. 27,
Issue. 3,
p.
557.
Baker, R. C.
1971.
A diophantine problem on groups. III.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 70,
Issue. 1,
p.
31.
Edwards, R.E.
1972.
Inequalities related to those of Hausdorff-Young.
Bulletin of the Australian Mathematical Society,
Vol. 6,
Issue. 2,
p.
185.
Brown, Gavin
and
Moran, William
1974.
On orthogonality of Riesz products.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 76,
Issue. 1,
p.
173.
Graham, Colin C.
1974.
A Riesz product proof of the Wiener-Pitt theorem.
Proceedings of the American Mathematical Society,
Vol. 44,
Issue. 2,
p.
312.
Brown, Gavin
and
Moran, William
1975.
Coin tossing and powers of singular measures.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 77,
Issue. 2,
p.
349.
Graham, Colin C.
1977.
Functional calculus and positive-definite functions.
Transactions of the American Mathematical Society,
Vol. 231,
Issue. 1,
p.
215.
Brown, Gavin
1978.
Integrability of Fourier transforms under an ergodic hypothesis.
Journal of the Australian Mathematical Society,
Vol. 26,
Issue. 2,
p.
129.
Maclean, Alan
1978.
Products of singular continuous measures.
Proceedings of the American Mathematical Society,
Vol. 68,
Issue. 1,
p.
85.
Ritter, G.
1979.
On Kakutani's dichotomy theorem for infinite products of not necessarily independent functions.
Mathematische Annalen,
Vol. 239,
Issue. 1,
p.
35.
Ritter, G.
1979.
On dichotomy of Riesz products.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 85,
Issue. 1,
p.
79.
Oberlin, Daniel M.
1979.
Singular measures andl p Fourier transforms.
Israel Journal of Mathematics,
Vol. 34,
Issue. 1-2,
p.
115.
MacLean, Alan
1980.
Modifications of Fourier transforms and singular continuous measures.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 87,
Issue. 3,
p.
383.
Janssen, Arnold
1980.
Charakterisierung stetiger Faltungshalbgruppen durch das L�vy-Ma�.
Mathematische Annalen,
Vol. 246,
Issue. 3,
p.
233.
Bachelis, Gregory F.
1983.
Radical Banach Algebras and Automatic Continuity.
Vol. 975,
Issue. ,
p.
301.
Hewitt, Edwin
and
Koshi, Shozo
1983.
Orderings in locally compact Abelian groups and the theorem of F. and M. Riesz.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 93,
Issue. 3,
p.
441.
Ritter, David L.
1986.
Most Riesz product measures are 𝐿^{𝑝}-improving.
Proceedings of the American Mathematical Society,
Vol. 97,
Issue. 2,
p.
291.
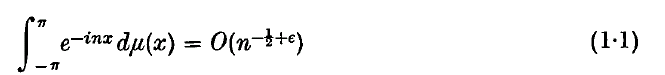
 For general locally compact Abelian groups, no exact analogue of (1 · 1) seems possible, as the character group may admit no natural order. However, it makes good sense to ask if μ* μ is absolutely continuous and has pth power integrable derivative. We will construct continuous singular measures μ on all non-discrete locally compact Abelian groups G such that μ * μ is a absolutely continuous and for which the Lebesgue–Radon–Nikodým derivative of μ * μ is in,
For general locally compact Abelian groups, no exact analogue of (1 · 1) seems possible, as the character group may admit no natural order. However, it makes good sense to ask if μ* μ is absolutely continuous and has pth power integrable derivative. We will construct continuous singular measures μ on all non-discrete locally compact Abelian groups G such that μ * μ is a absolutely continuous and for which the Lebesgue–Radon–Nikodým derivative of μ * μ is in,  for all real p > 1.
for all real p > 1.

