Some numerical results on the characters of exceptional Weyl groups
Published online by Cambridge University Press: 24 October 2008
Extract
1. Let V be an l-dimensional real vector space and let W be a finite subgroup of GL(V) generated by reflexions such that the space of W-invariant vectors in V is zero. Then W acts naturally on the symmetric algebra S of V preserving the natural grading 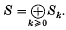 . LetI be the ideal in S generated by the w-invariant elements in
. LetI be the ideal in S generated by the w-invariant elements in 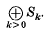 . The quotient algebra
. The quotient algebra  inherits the W-action and also a grading
inherits the W-action and also a grading
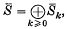
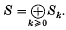 is the image of Sk under S → S̅. It is well known that the W-module S̅ is isomorphic to the regular representation of W (see (3), ch. v, 5·2); in particular, S̅k = 0 for large k. (More precisely, S̅k = 0 for k > ν, where ν is the number of reflexions in W.)If ρ is an irreducible character of W, we denote by nk(ρ) the multiplicity of ρ in the W- module S̅k. The sequence n(ρ) = (n0(ρ), n1(ρ), n2(ρ), …) is an interesting invariant of the character ρ For example, in the study of unipotent classes in semisimple groups, one encounters the following question: what is the smallest k for which nk(ρ) 4= 0 (with ρ as above) and, then, what is nk(ρ) Also, the polynomial in q
is the image of Sk under S → S̅. It is well known that the W-module S̅ is isomorphic to the regular representation of W (see (3), ch. v, 5·2); in particular, S̅k = 0 for large k. (More precisely, S̅k = 0 for k > ν, where ν is the number of reflexions in W.)If ρ is an irreducible character of W, we denote by nk(ρ) the multiplicity of ρ in the W- module S̅k. The sequence n(ρ) = (n0(ρ), n1(ρ), n2(ρ), …) is an interesting invariant of the character ρ For example, in the study of unipotent classes in semisimple groups, one encounters the following question: what is the smallest k for which nk(ρ) 4= 0 (with ρ as above) and, then, what is nk(ρ) Also, the polynomial in q
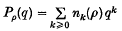
can sometimes be interpreted as the dimension of an irreducible representation of a Chevalley group over the field with q elements. For these reasons it seems desirable to describe explicitly the sequence n(ρ) (or, equivalently, the polynomial Pρ(q)) for the various irreducible characters ρ of W. When W is a Weyl group of type Al, this is contained in the work of Steinberg (8); in the case where W is a Weyl group of type Bl or Dl this is done in (7),§2.
Information
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 84 , Issue 3 , November 1978 , pp. 417 - 426
- Copyright
- Copyright © Cambridge Philosophical Society 1978
References
Referencess
- 37
- Cited by

