Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Byott, Nigel
1991.
On Galois isomorphisms between ideals in extensions of local fields.
Manuscripta Mathematica,
Vol. 73,
Issue. 1,
p.
289.
Aiba, Akira
2003.
Artin–Schreier extensions and Galois module structure.
Journal of Number Theory,
Vol. 102,
Issue. 1,
p.
118.
Huynh, Duc Van
2014.
Artin–Schreier extensions and generalized associated orders.
Journal of Number Theory,
Vol. 136,
Issue. ,
p.
28.


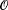
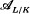 of the extension
of the extension 
