Article contents
Spherically symmetric differential equations, the rotation group, and tensor spherical functions
Published online by Cambridge University Press: 24 October 2008
Abstract
In this paper a scheme is developed for handling tensor partial differential equations having spherical symmetry. The basic technique is that of Gelfand and Shapiro ((2), §8) by which tensor fields defined on a sphere give rise to scalar fields defined on the rotation group 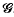 . These fields may be expanded as series of functions
. These fields may be expanded as series of functions  , where
, where 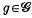 , m is fixed and the matrices Tl(g) form a 21 + 1 dimensional irreducible representation of
, m is fixed and the matrices Tl(g) form a 21 + 1 dimensional irreducible representation of 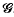 .
.
Spherically symmetric operations, such as covariant differentiation of tensors and the contraction of tensors with other spherically symmetric tensor fields, are shown to act in a particularly simple way on the terms of the series mentioned above: terms with given l, n are transformed into others with the same values of l, n. That this must be so follows from Schur's Lemma and the fact that for each m and l the functions  form a basis for an invariant subspace of functions on
form a basis for an invariant subspace of functions on 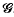 of dimension 2l + 1 in which an irreducible representation of
of dimension 2l + 1 in which an irreducible representation of 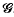 acts. Explicit formulae for the results of such operations are presented.
acts. Explicit formulae for the results of such operations are presented.
The results are used to show the existence of scalar potentials for tensors of all ranks and the results for tensors of the second rank are shown to be closely related to those recently obtained by Backus(1).
This work is intended for application in geophysics and other fields where spherical symmetry plays an important role. Since workers in these fields may not be familiar with quantum theory, some matter in sections 2–5 has been included in spite of the fact that it is well known in the quantum theory of angular momentum.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 65 , Issue 1 , January 1969 , pp. 157 - 175
- Copyright
- Copyright © Cambridge Philosophical Society 1969
References
REFERENCES
- 29
- Cited by


