Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Gillis, J.
and
Kaufman, B.
1962.
The stability of a rotating viscous jet.
Quarterly of Applied Mathematics,
Vol. 19,
Issue. 4,
p.
301.
Gillis, J.
and
Suh, K. S.
1962.
Stability of a Rotating Liquid Column.
The Physics of Fluids,
Vol. 5,
Issue. 10,
p.
1149.
Ross, Dieter K.
1970.
The stability of a column of liquid to torsional oscillations.
Zeitschrift für angewandte Mathematik und Physik ZAMP,
Vol. 21,
Issue. 1,
p.
137.
Ross, Dieter K.
1974.
Stability of an annulus of rotating liquid to plane disturbances.
The Physics of Fluids,
Vol. 17,
Issue. 6,
p.
1119.
Bauer, Helmut F.
1981.
Freie Schwingungen nichtmischbarer Flüssigkeiten im rotierenden Kreiszylinder unter Berücksichtigung der Oberflächenspannungen.
Forschung im Ingenieurwesen,
Vol. 47,
Issue. 6,
p.
190.
Da-Riva, I.
1981.
Applications of Space Developments.
p.
69.
Pimputkar, Sudheer M.
and
Ostrach, Simon
1981.
Convective effects in crystals grown from melt.
Journal of Crystal Growth,
Vol. 55,
Issue. 3,
p.
614.
Bauer, Helmut F.
1982.
Rotating finite liquid systems under zero-gravity.
Forschung im Ingenieurwesen,
Vol. 48,
Issue. 6,
p.
169.
Meseguer, J.
1983.
The breaking of axisymmetric slender liquid bridges.
Journal of Fluid Mechanics,
Vol. 130,
Issue. -1,
p.
123.
Bauer, Helmut F.
1984.
Surface- and interface oscillations of a rotating viscous liquid column of immiscible liquids.
Forschung im Ingenieurwesen,
Vol. 50,
Issue. 1,
p.
21.
Bauer, H. F.
1984.
Natural Damped Frequencies of an Infinitely Long Column of Immiscible Viscous Liquids.
ZAMM - Journal of Applied Mathematics and Mechanics / Zeitschrift für Angewandte Mathematik und Mechanik,
Vol. 64,
Issue. 11,
p.
475.
Langbein, Dieter
1986.
Materials Sciences in Space.
p.
401.
Bauer, Helmut F.
1986.
Surface- and interface oscillations of a rotating viscoelastic liquid column of immiscible liquids.
ZAMP Zeitschrift f�r angewandte Mathematik und Physik,
Vol. 37,
Issue. 4,
p.
514.
Bauer, H. F.
1989.
Damped response of an axially excited rotating liquid bridge in zero-gravity.
Acta Mechanica,
Vol. 79,
Issue. 3-4,
p.
295.
Bauer, Helmut F.
1989.
Response of a rotating finite annular liquid layer to axial excitation.
Forschung im Ingenieurwesen,
Vol. 55,
Issue. 4,
p.
120.
Bauer, H. F.
1990.
Response of differently axially excited spinning liquid columns.
Acta Mechanica,
Vol. 84,
Issue. 1-4,
p.
155.
Bauer, H. F.
1991.
Axi-symmetric natural frequencies and response of a spinning liquid column under strong surface tension.
Acta Mechanica,
Vol. 90,
Issue. 1-4,
p.
21.
Bauer, H. F.
1992.
Natural damped frequencies and axial response of a rotating finite viscous liquid column.
Acta Mechanica,
Vol. 93,
Issue. 1-4,
p.
29.
Bauer, Helmut F.
and
Kammerer, Lothar
1993.
Natural frequencies and axial response of a spinning liquid layer in a micro-gravity field.
ZAMP Zeitschrift f�r angewandte Mathematik und Physik,
Vol. 44,
Issue. 2,
p.
348.
Bauer, Helmut F.
1993.
Natural damped frequencies of a rotating viscous cylindrical liquid layer.
Forschung im Ingenieurwesen,
Vol. 59,
Issue. 10,
p.
217.
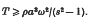 . This critical value is higher than that required for stability of the similar motion of a nonviscous liquid, but is otherwise independent of the coefficient of viscosity. The rate of development of instability when T is less than the critical value is also studied. Some numerical results are given.
. This critical value is higher than that required for stability of the similar motion of a nonviscous liquid, but is otherwise independent of the coefficient of viscosity. The rate of development of instability when T is less than the critical value is also studied. Some numerical results are given.