Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Luo, Jun Jason
and
Lau, Ka-Sing
2013.
Lipschitz equivalence of self-similar sets and hyperbolic boundaries.
Advances in Mathematics,
Vol. 235,
Issue. ,
p.
555.
LUO, JUN JASON
and
LIU, JING-CHENG
2016.
ON THE CLASSIFICATION OF FRACTAL SQUARES.
Fractals,
Vol. 24,
Issue. 01,
p.
1650008.
Zhang, Yanfang
2016.
Connectedness of invariant sets of graph-directed IFS.
Wuhan University Journal of Natural Sciences,
Vol. 21,
Issue. 5,
p.
445.
Cristea, Ligia L.
and
Steinsky, Bertran
2017.
Mixed labyrinth fractals.
Topology and its Applications,
Vol. 229,
Issue. ,
p.
112.
LIU, JING-CHENG
LUO, JUN JASON
and
TANG, KE
2017.
CONNECTEDNESS OF SELF-AFFINE SETS WITH PRODUCT DIGIT SETS.
Fractals,
Vol. 25,
Issue. 06,
p.
1750053.
Zhang, Yuan
2017.
Topological structure of a class of planar self-affine sets.
Journal of Mathematical Analysis and Applications,
Vol. 446,
Issue. 2,
p.
1524.
Ruan, Huo-Jun
and
Wang, Yang
2017.
Topological invariants and Lipschitz equivalence of fractal squares.
Journal of Mathematical Analysis and Applications,
Vol. 451,
Issue. 1,
p.
327.
Zhu, Yunjie
and
Yang, Yamin
2018.
Lipschitz equivalence of self-similar sets with two-state neighbor automaton.
Journal of Mathematical Analysis and Applications,
Vol. 458,
Issue. 1,
p.
379.
Bandt, Christoph
and
Mekhontsev, Dmitry
2018.
Elementary fractal geometry. New relatives of the Sierpiński gasket.
Chaos: An Interdisciplinary Journal of Nonlinear Science,
Vol. 28,
Issue. 6,
Fu, Xiaoye
and
Gabardo, Jean‐Pierre
2019.
Decomposition of integral self‐affine multi‐tiles.
Mathematische Nachrichten,
Vol. 292,
Issue. 6,
p.
1304.
CRISTEA, LIGIA L.
and
SURER, PAUL
2019.
TRIANGULAR LABYRINTH FRACTALS.
Fractals,
Vol. 27,
Issue. 08,
p.
1950131.
Xi, Lifeng
2020.
Differentiable points of Sierpinski-like sponges.
Advances in Mathematics,
Vol. 361,
Issue. ,
p.
106936.
ZHANG, YAN-FANG
2020.
A LOWER BOUND OF TOPOLOGICAL HAUSDORFF DIMENSION OF FRACTAL SQUARES.
Fractals,
Vol. 28,
Issue. 06,
p.
2050115.
Ma, Ji-hua
and
Zhang, Yan-fang
2020.
Topological Hausdorff dimension of fractal squares and its application to Lipschitz classification.
Nonlinearity,
Vol. 33,
Issue. 11,
p.
6053.
Xiao, Jian-Ci
2021.
Fractal squares with finitely many connected components
*
.
Nonlinearity,
Vol. 34,
Issue. 4,
p.
1817.
Huang, Liang-yi
and
Rao, Hui
2021.
A dimension drop phenomenon of fractal cubes.
Journal of Mathematical Analysis and Applications,
Vol. 497,
Issue. 2,
p.
124918.
ZHU, YUNJIE
and
RAO, HUI
2021.
LIPSCHITZ EQUIVALENCE OF SELF-SIMILAR SETS AND FINITE-STATE AUTOMATON.
Fractals,
Vol. 29,
Issue. 08,
Liang, Zhen
Miao, Jun Jie
and
Ruan, Huo-Jun
2022.
Gap sequences and Topological properties of Bedford–McMullen sets*
.
Nonlinearity,
Vol. 35,
Issue. 8,
p.
4043.
Luo, Jun
Rao, Hui
and
Xiong, Ying
2022.
Every component of a fractal square is a Peano continuum.
Topology and its Applications,
Vol. 322,
Issue. ,
p.
108271.
Das, Sukanta Kumar
Mishra, Jibitesh
and
Nayak, Soumya Ranjan
2022.
A mathematical model for generation of interactive fractals using a fourth order fractal square: A case study.
Journal of Interdisciplinary Mathematics,
Vol. 25,
Issue. 7,
p.
1979.


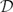 ⊊ {0,1,. . .,
⊊ {0,1,. . .,