Published online by Cambridge University Press: 12 July 2019
May the triforce be the 3-uniform hypergraph on six vertices with edges {123′, 12′3, 1′23}. We show that the minimum triforce density in a 3-uniform hypergraph of edge density δ is δ4–o(1) but not O(δ4).
Let M(δ) be the maximum number such that the following holds: for every ∊ > 0 and 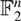 $G = {\mathbb{F}}_2^n$ with n sufficiently large, if A ⊆ G × G with A ≥ δ|G|2, then there exists a nonzero “popular difference” d ∈ G such that the number of “corners” (x, y), (x + d, y), (x, y + d) ∈ A is at least (M(δ)–∊)|G|2. As a corollary via a recent result of Mandache, we conclude that M(δ) = δ4–o(1) and M(δ) = ω(δ4).
$G = {\mathbb{F}}_2^n$ with n sufficiently large, if A ⊆ G × G with A ≥ δ|G|2, then there exists a nonzero “popular difference” d ∈ G such that the number of “corners” (x, y), (x + d, y), (x, y + d) ∈ A is at least (M(δ)–∊)|G|2. As a corollary via a recent result of Mandache, we conclude that M(δ) = δ4–o(1) and M(δ) = ω(δ4).
On the other hand, for 0 < δ < 1/2 and sufficiently large N, there exists A ⊆ [N]3 with |A| ≥ δN3 such that for every d ≠ 0, the number of corners (x, y, z), (x + d, y, z), (x, y + d, z), (x, y, z + d) ∈ A is at most δc log(1/δ)N3. A similar bound holds in higher dimensions, or for any configuration with at least 5 points or affine dimension at least 3.
Supported by a Packard Fellowship and NSF Career Award DMS-1352121
Supported by NSF Award DMS-1764176, and the MIT Solomon Buchsbaum Fund