No CrossRef data available.
Published online by Cambridge University Press: 24 October 2008
0·0. Let G be an infinite compact abelian group with dual X. Parseval's identity shows that if f ∈ C(G) and ω ∈ l∞(X) then  . Edwards has shown in (2) that L2(G) here cannot, in general, be replaced by any smaller Lp(G) space. Precisely: there exist f ∈ C(G) and ω: X → {± 1} such that
. Edwards has shown in (2) that L2(G) here cannot, in general, be replaced by any smaller Lp(G) space. Precisely: there exist f ∈ C(G) and ω: X → {± 1} such that 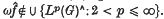 . We strengthen this result by showing much more can be said about the summability of the Fourier series of f than
. We strengthen this result by showing much more can be said about the summability of the Fourier series of f than 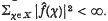 . For example, when G is the circle group, f can be chosen to also satisfy
. For example, when G is the circle group, f can be chosen to also satisfy
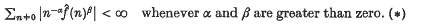
The functions introduced here and called darts, generalize this type of series condition.