No CrossRef data available.
Article contents
Uniformly asymptotic expansions for an integral with a large and a small parameter
Published online by Cambridge University Press: 24 October 2008
Abstract
The integral

involves a large real parameter N and a small real parameter ∈. Its asymptotic behaviour is non-uniform when N → ∞ and ∈ → 0. Thus, when ∈ > 0 is kept fixed and N → ∞, the integral decays exponentially at a rate depending on ∈; when ∈ → 0 the integral tends to
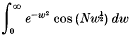
which decays algebraically when N → ∞. It is shown that several distinct uniformly asymptotic expansions can be obtained which each involve an infinite set of functions of the combination 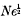 Certain related integrals are also treated.
Certain related integrals are also treated.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 101 , Issue 2 , March 1987 , pp. 349 - 362
- Copyright
- Copyright © Cambridge Philosophical Society 1987


