No CrossRef data available.
Published online by Cambridge University Press: 23 March 2017
We consider the notion of a free resolution. In general, a free resolution can be of any length depending on the group ring under investigation. The metacyclic groups 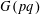 $G(pq)$ however admit periodic resolutions. In the particular case of
$G(pq)$ however admit periodic resolutions. In the particular case of  $G(21)$ it is possible to achieve a fully diagonalized resolution. In order to achieve a diagonal resolution, we obtain a complete list of indecomposable modules over
$G(21)$ it is possible to achieve a fully diagonalized resolution. In order to achieve a diagonal resolution, we obtain a complete list of indecomposable modules over 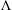 $\unicode[STIX]{x1D6EC}$ . Such a list aids the decomposition of the augmentation ideal (the first syzygy) into a direct sum of indecomposable modules. Therefore, we are able to achieve a diagonalized map here. From this point it is possible to decompose all of the remaining syzygies in terms of indecomposable modules, leaving a diagonal resolution.
$\unicode[STIX]{x1D6EC}$ . Such a list aids the decomposition of the augmentation ideal (the first syzygy) into a direct sum of indecomposable modules. Therefore, we are able to achieve a diagonalized map here. From this point it is possible to decompose all of the remaining syzygies in terms of indecomposable modules, leaving a diagonal resolution.