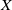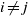Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Guirao, Antonio J.
Montesinos, Vicente
and
Zizler, Václav
2016.
Open Problems in the Geometry and Analysis of Banach Spaces.
p.
125.
Swanepoel, Konrad J.
2018.
New Trends in Intuitive Geometry.
Vol. 27,
Issue. ,
p.
407.
Russo, Tommaso
2019.
A note on symmetric separation in Banach spaces.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 113,
Issue. 4,
p.
3649.
Koszmider, Piotr
and
Wark, Hugh
2022.
Large Banach spaces with no infinite equilateral sets.
Bulletin of the London Mathematical Society,
Vol. 54,
Issue. 6,
p.
2066.
Koszmider, Piotr
and
Ryduchowski, Kamil
2023.
Equilateral and separated sets in some Hilbert generated Banach spaces.
Proceedings of the American Mathematical Society,
Koszmider, Piotr
2024.
BANACH SPACES IN WHICH LARGE SUBSETS OF SPHERES CONCENTRATE.
Journal of the Institute of Mathematics of Jussieu,
Vol. 23,
Issue. 2,
p.
737.
Kobos, Tomasz
and
Swanepoel, Konrad
2025.
Equilateral dimension of the planar Banach–Mazur compactum.
Proceedings of the American Mathematical Society,