Article contents
INTERMEDIATE SUMS ON POLYHEDRA II: BIDEGREE AND POISSON FORMULA
Published online by Cambridge University Press: 29 February 2016
Abstract
We continue our study of intermediate sums over polyhedra, interpolating between integrals and discrete sums, which were introduced by Barvinok [Computing the Ehrhart quasi-polynomial of a rational simplex. Math. Comp. 75 (2006), 1449–1466]. By well-known decompositions, it is sufficient to consider the case of affine cones 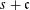 $s+\mathfrak{c}$ , where
$s+\mathfrak{c}$ , where  $s$ is an arbitrary real vertex and
$s$ is an arbitrary real vertex and  $\mathfrak{c}$ is a rational polyhedral cone. For a given rational subspace
$\mathfrak{c}$ is a rational polyhedral cone. For a given rational subspace 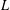 $L$ , we define the intermediate generating functions
$L$ , we define the intermediate generating functions  $S^{L}(s+\mathfrak{c})(\unicode[STIX]{x1D709})$ by integrating an exponential function over all lattice slices of the affine cone
$S^{L}(s+\mathfrak{c})(\unicode[STIX]{x1D709})$ by integrating an exponential function over all lattice slices of the affine cone 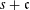 $s+\mathfrak{c}$ parallel to the subspace
$s+\mathfrak{c}$ parallel to the subspace 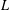 $L$ and summing up the integrals. We expose the bidegree structure in parameters
$L$ and summing up the integrals. We expose the bidegree structure in parameters  $s$ and
$s$ and  $\unicode[STIX]{x1D709}$ , which was implicitly used in the algorithms in our papers [Computation of the highest coefficients of weighted Ehrhart quasi-polynomials of rational polyhedra. Found. Comput. Math.12 (2012), 435–469] and [Intermediate sums on polyhedra: computation and real Ehrhart theory. Mathematika59 (2013), 1–22]. The bidegree structure is key to a new proof for the Baldoni–Berline–Vergne approximation theorem for discrete generating functions [Local Euler–Maclaurin expansion of Barvinok valuations and Ehrhart coefficients of rational polytopes. Contemp. Math.452 (2008), 15–33], using the Fourier analysis with respect to the parameter
$\unicode[STIX]{x1D709}$ , which was implicitly used in the algorithms in our papers [Computation of the highest coefficients of weighted Ehrhart quasi-polynomials of rational polyhedra. Found. Comput. Math.12 (2012), 435–469] and [Intermediate sums on polyhedra: computation and real Ehrhart theory. Mathematika59 (2013), 1–22]. The bidegree structure is key to a new proof for the Baldoni–Berline–Vergne approximation theorem for discrete generating functions [Local Euler–Maclaurin expansion of Barvinok valuations and Ehrhart coefficients of rational polytopes. Contemp. Math.452 (2008), 15–33], using the Fourier analysis with respect to the parameter  $s$ and a continuity argument. Our study also enables a forthcoming paper, in which we study intermediate sums over multi-parameter families of polytopes.
$s$ and a continuity argument. Our study also enables a forthcoming paper, in which we study intermediate sums over multi-parameter families of polytopes.
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © University College London 2016
References
- 1
- Cited by

