Published online by Cambridge University Press: 26 February 2010
The following theorem has been proved by Bambah, Rogers and Zassenhaus [1],
Theorem A. Let K be a closed convex domain with a centre. Let
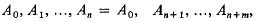
be points such that:
(i) the polygon A0 A1 … An is a Jordan polygon bounding a closed domain π of area a(π);
(ii) for each r, 0 ≤ r ≤ n, there is a point common to K + An-1 and K + Ar;
(iii) the points An+1, …. An+m are in the interior of π;
(iv) for each point X of π, there exists an Ar, 1 ≤ r ≤ n + m, such that X ∈ K + Ar and the line segment XAr is in π. Then
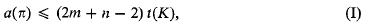
where t(K) is the area of the largest triangle contained in K.