Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Duan, Yu
Liao, Jia-Feng
and
Sun, Xin
2018.
An exact estimate result for singularp-Laplacian equations with sign-changing potential.
Computers & Mathematics with Applications,
Vol. 76,
Issue. 8,
p.
2022.
Duan, Yu
Li, Hong-Ying
and
Sun, Xin
2021.
Uniqueness of positive solutions for a class of p-Kirchhoff type problems with singularity.
Rocky Mountain Journal of Mathematics,
Vol. 51,
Issue. 5,
Chata, Juan Carlos Ortiz
Pimenta, Marcos T.O.
and
de León, Sergio Segura
2023.
Existence of solutions to a 1-Laplacian problem with a concave-convex nonlinearity.
Journal of Mathematical Analysis and Applications,
Vol. 525,
Issue. 2,
p.
127149.
Wang, Chen-Xi
and
Suo, Hong-Min
2024.
Infinitely many positive solutions for p-Laplacian equations with singular and critical growth terms.
Boundary Value Problems,
Vol. 2024,
Issue. 1,
Wu, Zijian
and
Chen, Haibo
2024.
Multiplicity of Solutions for a singular Problem Involving the n-Laplacian.
Qualitative Theory of Dynamical Systems,
Vol. 23,
Issue. 2,
Pimenta, Marcos T. O.
Carranza, Yino B. C.
and
Figueiredo, Giovany M.
2024.
Existence results for the 1-Laplacian problem with a critical concave–convex nonlinearity.
Journal of Fixed Point Theory and Applications,
Vol. 26,
Issue. 4,
de Souza Medeiros, Aldo Henrique
Pereira, Gilberto Assis
and
Silva, Jeferson Camilo
2025.
Multiplicity of solutions for singular fractional $$1$$-Laplacian problems: an approximation approach.
Annali di Matematica Pura ed Applicata (1923 -),

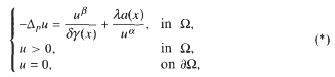
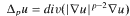 , 1 <
, 1 < 