Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-27T06:36:44.343Z Has data issue: false hasContentIssue false
  • English
  • Français

A CLASS OF IRREDUCIBLE POLYNOMIALS ASSOCIATED WITH PRIME DIVISORS OF VALUES OF CYCLOTOMIC POLYNOMIALS

Part of: Polynomials and matrices Multiplicative number theory Algebraic number theory: global fields Sequences and sets General field theory

Published online by Cambridge University Press:  14 August 2019

Michael Filaseta
Michael Filaseta*
Affiliation:
Department of Mathematics, University of South Carolina, Columbia, SC 29208, U.S.A. email filaseta@math.sc.edu
Get access

Abstract

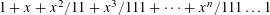We prove that for every sufficiently large integer $n$, the polynomial $1+x+x^{2}/11+x^{3}/111+\cdots +x^{n}/111\ldots 1$ is irreducible over the rationals, where the coefficient of 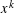$x^{k}$ for 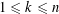$1\leqslant k\leqslant n$ is the reciprocal of the decimal number consisting of 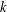$k$ digits which are each 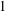$1$. Similar results following from the same techniques are discussed.

MSC classification

Primary: 11R09: Polynomials (irreducibility, etc.)
Secondary: 11C08: Polynomials 11B83: Special sequences and polynomials 11N05: Distribution of primes 12E05: Polynomials (irreducibility, etc.)
Type
Research Article
Information
Mathematika , Volume 65 , Issue 4 , 2019 , pp. 1033 - 1037
Copyright
Copyright © University College London 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Apostol, T. M., Resultants of cyclotomic polynomials. Proc. Amer. Math. Soc. 24 1970, 462.10.1090/S0002-9939-1970-0251010-XGoogle Scholar
Dickson, L. E., History of the Theory of Numbers, Vol. I: Divisibility and Primality, Chelsea (New York, 1966).Google Scholar
Dresden, G., Resultants of cyclotomic polynomials. Rocky Mountain J. Math. 42 2012, 1461–1469.10.1216/RMJ-2012-42-5-1461Google Scholar
Dumas, G., Sur quelques cas d’irréductibilité des polynômes à coefficients rationnels. J. Math. Pures Appl. (9) 2 1906, 191–258.Google Scholar
Filaseta, M., Coverings of the integers associated with an irreducibility theorem of A. Schinzel. In Number Theory for the Millennium, II (Urbana, IL, 2000), A. K. Peters (Natick, MA, 2002), 1–24.Google Scholar
Halberstam, H. and Richert, H.-E., Sieve Methods, Dover (Mineola, NY, 2011). Originally published by Academic Press (London Mathematical Society Monographs 4 (1974)).Google Scholar
Stewart, C. L., On divisors of Lucas and Lehmer numbers. Acta Math. 211 2013, 291–314.10.1007/s11511-013-0105-yGoogle Scholar