Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Lev, Vsevolod F.
2008.
The rectifiability threshold in abelian groups.
Combinatorica,
Vol. 28,
Issue. 4,
p.
491.
Grynkiewicz, David J.
2013.
Structural Additive Theory.
Vol. 30,
Issue. ,
p.
299.
Jung, Peter
and
Walk, Philipp
2015.
Compressed Sensing and its Applications.
p.
283.
Walk, Philipp
Jung, Peter
and
Pfander, Götz E.
2017.
On the stability of sparse convolutions.
Applied and Computational Harmonic Analysis,
Vol. 42,
Issue. 1,
p.
117.
Grochow, Joshua
2018.
New applications of the polynomial method: The cap set conjecture and beyond.
Bulletin of the American Mathematical Society,
Vol. 56,
Issue. 1,
p.
29.
Wooley, Trevor D.
2025.
Combinatorial and Additive Number Theory VI.
Vol. 464,
Issue. ,
p.
461.

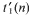 of classes of such cardinality
of classes of such cardinality 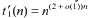 .
.