Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-13T05:45:49.330Z Has data issue: false hasContentIssue false
  • English
  • Français

CONTROLLING LIPSCHITZ FUNCTIONS

Part of: Discrete geometry Low-dimensional topology

Published online by Cambridge University Press:  02 August 2018

Andrey Kupavskii ,
János Pach  and
Gábor Tardos
Andrey Kupavskii
Affiliation:
EPFL, Lausanne, Switzerland MIPT, Moscow, Russia email kupavskii@ya.ru
János Pach
Affiliation:
EPFL, Lausanne, Switzerland Rényi Institute, Budapest, Hungary email pach@cims.nyu.edu
Gábor Tardos
Affiliation:
Rényi Institute, Budapest, Hungary Central European University, Budapest, Hungary email tardos@renyi.hu
Get access

Abstract

Given any positive integers $m$ and 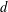$d$, we say a sequence of points 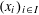$(x_{i})_{i\in I}$ in 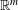$\mathbb{R}^{m}$ is Lipschitz-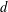$d$-controlling if one can select suitable values 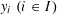$y_{i}\;(i\in I)$ such that for every Lipschitz function $f\,:\,\mathbb{R}^{m}\,\rightarrow \,\mathbb{R}^{d}$ there exists 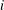$i$ with 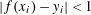$|f(x_{i})\,-\,y_{i}|\,<\,1$. We conjecture that for every 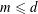$m\leqslant d$, a sequence 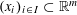$(x_{i})_{i\in I}\subset \mathbb{R}^{m}$ is $d$-controlling if and only if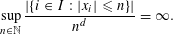

$$\begin{eqnarray}\displaystyle \sup _{n\in \mathbb{N}}\frac{|\{i\in I:|x_{i}|\leqslant n\}|}{n^{d}}=\infty . & & \displaystyle \nonumber\end{eqnarray}$$
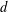We prove that this condition is necessary and a slightly stronger one is already sufficient for the sequence to be $d$-controlling. We also prove the conjecture for $m=1$.

MSC classification

Primary: 52C17: Packing and covering in $n$ dimensions
Secondary: 57M20: Two-dimensional complexes
Type
Research Article
Information
Mathematika , Volume 64 , Issue 3 , 2018 , pp. 898 - 910
Copyright
Copyright © University College London 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bang, Th., On covering by parallel-strips. Mat. Tidsskr. B. 1950 1950, 49–53.Google Scholar
Bang, Th., A solution of the “plank problem”. Proc. Amer. Math. Soc. 2 1951, 990–993.Google Scholar
Brass, P., Moser, W. and Pach, J., Research Problems in Discrete Geometry, Springer (Heidelberg, 2005).Google Scholar
Erdős, P. and Pach, J., On a problem of L. Fejes Tóth. Discrete Math. 30(2) 1980, 103–109.Google Scholar
Fejes Tóth, L., Remarks on the dual of Tarski’s plank problem. Mat. Lapok (N.S.) 25 1974, 13–20 (in Hungarian).Google Scholar
Kupavskii, A. and Pach, J., Simultaneous approximation of polynomials. In Discrete and Computational Geometry and Graphs (Lecture Notes in Computer Science 9943 ) (eds Akiyama, J., Ito, H., Sakai, T. and Uno, Y.), Springer (Cham, 2016), 193–203.Google Scholar
Makai, E. Jr. and Pach, J., Controlling function classes and covering Euclidean space. Studia Sci. Math. Hungar. 18 1983, 435–459.Google Scholar
McFarland, A., McFarland, J. and Smith, J. T. (eds), Alfred Tarski: Early Work in Poland–Geometry and Teaching, Birkhäuser/Springer (New York, 2014); with a bibliographic supplement, Foreword by Ivor Grattan–Guinness.Google Scholar
Moese, H., Przyczynek do problemu A. Tarskiego: “O stopniu równowaonosci wielokatów”. Parametr 2 1932, 305–309 (A contribution to the problem of A. Tarski, “On the degree of equivalence of polygons”).Google Scholar
Tarski, A., Uwagi o stopnii równowaznosci wielokatów. Parametr 2 1932, 310–314.Google Scholar