Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Brüdern, Jörg
1991.
Additive diophantine inequalities with mixed powers, III.
Journal of Number Theory,
Vol. 37,
Issue. 2,
p.
199.
Brüdern, J.
and
Cook, R. J.
1991.
On pairs of cubic Diophantine inequalities.
Mathematika,
Vol. 38,
Issue. 2,
p.
250.
Brüdern, Jörg
1993.
Séminaire de Théorie des Nombres, Paris, 1990–91.
p.
23.
Baker, R. C.
Brüdern, J.
and
Wooley, T. D.
1995.
Cubic Diophantine inequalities.
Mathematika,
Vol. 42,
Issue. 2,
p.
264.
Brüdern, Jörg
2001.
Cubic diophantine inequalities III.
Periodica Mathematica Hungarica,
Vol. 42,
Issue. 1-2,
p.
211.
Xue, Boqing
2014.
Diophantine inequality involving binary forms.
Frontiers of Mathematics in China,
Vol. 9,
Issue. 3,
p.
641.

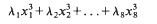
 the inequality
the inequality
 . In this note we improve this further, the progress being considerable.
. In this note we improve this further, the progress being considerable.