Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-29T02:48:27.094Z Has data issue: false hasContentIssue false
  • English
  • Français

A generalization of Ascoli's theorem

Published online by Cambridge University Press:  26 February 2010

J. D. Weston
J. D. Weston
Affiliation:
University of Durham, King's College, Newcastle upon Tyne.
Get access

Extract

In its simplest form, the theorem of Ascoli with which we are concerned is an extension of the Bolzano-Weierstrass theorem: it states that, if X and Y are bounded closed sets, of real or complex numbers, and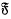is a sequence of equicontinuous functions mapping X into Y, then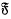has a uniformly convergent subsequence. As is well known, this result has played a fundamental part in the development of several theories; it has also been widely generalized, by processes of abstraction and localization, and a very useful version of the theorem runs as follows (cf. [4], 233–234):

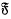(A) Suppose that X is a locally compact regular space, and that Y is a Hausdorff space whose topology is determined by a uniform structure. Let YX be the space of all functions that map X into Y, with the topology of locally uniform convergence with respect to. Then a closed setin YX is compact if, at each point x of X, (i) the set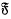(x) is relatively compact, in Y, and, (ii) 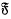is equicontinuous with respect to.

Type
Research Article
Information
Mathematika , Volume 6 , Issue 1 , June 1959 , pp. 19 - 24
Copyright
Copyright © University College London 1959

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1.Ascoli, G., “Le curve limite di una varietà data di curve”, Mem. Accad. Lincei (3), 18 (1883), 521–586.Google Scholar
2.Arzelà, C., “Sull' integrabilita delle equazioni differenziali ordinarie”, Mem. Accad. Bologna (5), 5 (1895–6), 257–270.Google Scholar
3.Saks, S. and Zygmund, A., Analytic functions (Warsaw, 1952).Google Scholar
4.Kelley, J. L., General topology (New York, 1955).Google Scholar
5.Gale, D., “Compact sets of functions and function rings”, Proc. American Math. Soc., 1 (1950), 303–308.CrossRefGoogle Scholar
6.Kelley, J. L., “The Tychonoff product theorem implies the axiom of choice”, Fundamenta Math., 37 (1950), 75–76.CrossRefGoogle Scholar
7.Bourbaki, N., Éléments de mathématique, X (Paris, 1949).Google Scholar