No CrossRef data available.
Article contents
Matrix transformations of series of orthogonal polynomials
Part of:
Geometric function theory
Published online by Cambridge University Press: 26 February 2010
Abstract
For a sequence of polynomials (Pn) orthonormal on the interval [−1, 1], we consider the sequence of transforms (gn) of the series 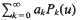 given by
given by 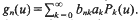 . We establish necessary and sufficient conditions on the matrix (bnk) for the sequence (gn) to converge uniformly on compact subsets of the interior of an appropriate ellipse to a function holomorphic on that interior.
. We establish necessary and sufficient conditions on the matrix (bnk) for the sequence (gn) to converge uniformly on compact subsets of the interior of an appropriate ellipse to a function holomorphic on that interior.
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © University College London 1995
References
1.Borwein, D. and Jakimovski, A.. Matrix transformations of power series. Proc. Amer. Math. Soc, 122 (1994), 511–523.CrossRefGoogle Scholar
2.Erdélyi, T.. Nikolskii-type inequalities of generalized polynomials and zeros of orthogonal polynomials. J. Approximation Theory, 67 (1991), 80–92.CrossRefGoogle Scholar
4.Lejà, M. F.. Sur la sommation des séries entières par la methode des moyennes. Bull. Sci. Math., 54 (1930), 239–245.Google Scholar
6.Luh, W.. Über die Nürlund-Summierbarkeit von Potenzreihen. Period. Math. Hungar., 5 (1974), 47–60.CrossRefGoogle Scholar
7.Luh, W.. Summierbarkeit von Potenzreihen-notwendige Bedingungen. Mitteilungen Math. Sem. Giessen, 113 (1974), 48–67.Google Scholar
8.Peyerimhoff, A.. Lectures on Summability, vol. 107. Springer-Verlag Lecture Notes in Mathematics (New York, 1969).Google Scholar
9.Stadtmüller, K.. Summability of power series by non-regular Nörlund methods. J. Approximation Theory, 68 (1992), 33–44.CrossRefGoogle Scholar
10.Stadtmüller, K. and Grosse-Erdmann, K. G.. Characterization of summability points of Nörlund methods. Trans. Amer. Math. Soc, 347 (1995), 2563–2579.Google Scholar

