Article contents
TRANSCENDENTAL SUMS RELATED TO THE ZEROS OF ZETA FUNCTIONS
Published online by Cambridge University Press: 01 August 2018
Abstract
While the distribution of the non-trivial zeros of the Riemann zeta function constitutes a central theme in Mathematics, nothing is known about the algebraic nature of these non-trivial zeros. In this article, we study the transcendental nature of sums of the form 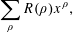 $$\begin{eqnarray}\mathop{\sum }_{\unicode[STIX]{x1D70C}}R(\unicode[STIX]{x1D70C})x^{\unicode[STIX]{x1D70C}},\end{eqnarray}$$
$$\begin{eqnarray}\mathop{\sum }_{\unicode[STIX]{x1D70C}}R(\unicode[STIX]{x1D70C})x^{\unicode[STIX]{x1D70C}},\end{eqnarray}$$ $\unicode[STIX]{x1D70C}$ of
$\unicode[STIX]{x1D70C}$ of  $\unicode[STIX]{x1D701}(s)$,
$\unicode[STIX]{x1D701}(s)$, 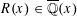 $R(x)\in \overline{\mathbb{Q}}(x)$ is a rational function over algebraic numbers and
$R(x)\in \overline{\mathbb{Q}}(x)$ is a rational function over algebraic numbers and 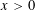 $x>0$ is a real algebraic number. In particular, we show that the function
$x>0$ is a real algebraic number. In particular, we show that the function  $$\begin{eqnarray}f(x)=\mathop{\sum }_{\unicode[STIX]{x1D70C}}\frac{x^{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}}\end{eqnarray}$$
$$\begin{eqnarray}f(x)=\mathop{\sum }_{\unicode[STIX]{x1D70C}}\frac{x^{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}}\end{eqnarray}$$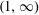 $(1,\infty )$, at most one of which is algebraic. The transcendence tools required for studying
$(1,\infty )$, at most one of which is algebraic. The transcendence tools required for studying 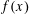 $f(x)$ in the range
$f(x)$ in the range 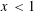 $x<1$ seem to be different from those in the range
$x<1$ seem to be different from those in the range  $x>1$. For
$x>1$. For 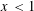 $x<1$, we have the following non-vanishing theorem: If for an integer
$x<1$, we have the following non-vanishing theorem: If for an integer  $d\geqslant 1$,
$d\geqslant 1$, 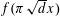 $f(\unicode[STIX]{x1D70B}\sqrt{d}x)$ has a rational zero in
$f(\unicode[STIX]{x1D70B}\sqrt{d}x)$ has a rational zero in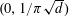 $(0,1/\unicode[STIX]{x1D70B}\sqrt{d})$, then
$(0,1/\unicode[STIX]{x1D70B}\sqrt{d})$, then  $$\begin{eqnarray}L^{\prime }(1,\unicode[STIX]{x1D712}_{-d})\neq 0,\end{eqnarray}$$
$$\begin{eqnarray}L^{\prime }(1,\unicode[STIX]{x1D712}_{-d})\neq 0,\end{eqnarray}$$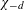 $\unicode[STIX]{x1D712}_{-d}$ is the quadratic character associated with the imaginary quadratic field
$\unicode[STIX]{x1D712}_{-d}$ is the quadratic character associated with the imaginary quadratic field 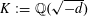 $K:=\mathbb{Q}(\sqrt{-d})$. Finally, we consider analogous questions for elements in the Selberg class. Our proofs rest on results from analytic as well as transcendental number theory.
$K:=\mathbb{Q}(\sqrt{-d})$. Finally, we consider analogous questions for elements in the Selberg class. Our proofs rest on results from analytic as well as transcendental number theory.
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © University College London 2018
Footnotes
Research of the second author was supported by an NSERC Discovery grant and a Simons Fellowship.
References
- 4
- Cited by

