Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-29T04:18:40.407Z Has data issue: false hasContentIssue false
  • English
  • Français

High-Purity Germanium Technology for Gamma-Ray and X-Ray Spectroscopy

Published online by Cambridge University Press:  21 February 2011

L.S. Darken  and
C. E. Cox
L.S. Darken
Affiliation:
Oxford Instruments Inc., 601 Oak Ridge Turnpike, Oak Ridge, TN 37831-2650 USA
C. E. Cox
Affiliation:
Oxford Instruments Inc., 601 Oak Ridge Turnpike, Oak Ridge, TN 37831-2650 USA
Get access

Abstract

High-purity germanium (HPGe) for gamma-ray spectroscopy is a mature technology that continues to evolve. Detector size is continually increasing, allowing efficient detection of higher energy gamma rays and improving the count rate and minimum detectable activity for lower energy gamma rays. For low-energy X rays, entrance window thicknesses have been reduced to where they are comparable to those in Si(Li) detectors. While some limits to HPGe technology are set by intrinsic properties, the frontiers have historically been determined by the level of control over extrinsic properties. The point defects responsible for hole trapping are considered in terms of the “standard level” model for hole capture. This model originates in the observation that the magnitude and temperature dependence of the cross section for hole capture at many acceptors in germanium is exactly that obtained if all incident s-wave holes were captured. That is, the capture rate is apparently limited by the arrival rate of holes that can make an angular-momentum-conserving transition to a s ground state. This model can also be generalized to other materials, where it may serve as an upper limit for direct capture into the ground state for either electrons or holes. The capture cross section for standard levels σS.L. is given by

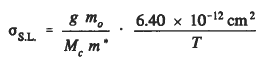
where g is the degeneracy of the ground state of the center after capture, divided by the degeneracy before capture. Mc is the number of equivalent extrema in the band structure for the carrier being captured, mo is the electronic mass, m* is the effective mass, and T is the temperature in degrees Kelvin.

Type
Research Article
Information
MRS Online Proceedings Library (OPL) , Volume 302: Symposium F – Semiconductors for Room-Temperature Radiation Detector Applications , 1993 , 31
Copyright
Copyright © Materials Research Society 1993

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

1. Teal, G.K. and Little, J.B., Phys. Rev. 78, 647 (1950).Google Scholar
2. Hall, R.N. in Semiconductor Materials for γ-Ray Detectors-Proceedings of the Meeting, W.L. Brown (BTL) and Wagner, S. (BNL) eds. (1966) p. 27.Google Scholar
3. Hall, R.N., IEEE Trans. Nuci. Sci. 21, 260 (1974).Google Scholar
4. Haller, E.E., Hansen, W.L., and Goulding, F.S., Adv. in Phys. 30, 93 (1981).Google Scholar
5. Darken, L.S., IEEE Trans. Nucl. Sci. NS–26, 324 (1979).Google Scholar
6. Pehl, R.H., Madden, N.W., Elliot, J.H., Raudorf, R.W., Trammell, R.C., and Darken, L.S. Jr., IEEE Trans. Nucl. Sci. NS–26, 321 (1979).CrossRefGoogle Scholar
7. Llacer, J., Haller, E.E., and Cordi, R.C., IEEE Trans. Nuci. Sci. NS–24 (1), 53 (1977).Google Scholar
8. Slapa, M., Chwaszczewska, J., Huth, G.C., and Jurkowski, J., Nucl. Instr. Meth 196, 575 (1982).Google Scholar
9. Barbi, N.C., and Lister, D.B., NBS Special Publication No. 604, 35 (1981).Google Scholar
10. Fink, R.W., NBS Special Publication No. 604, 5 (1981).Google Scholar
11. Steel, E.B., Microbeam Analysis 1986. Proc. 21st Annual Conf. Microbeam Analysis Soc. (1986), p. 439.Google Scholar
12. Zullinger, H.R., and Aitken, D.W., IEEE Trans. Nucl. Sci. NS–15, 466 (1968).Google Scholar
13. Zullinger, H.R. and Aitken, D.W., IEEE Trans. Nucl. Sci. NS–16, 47 (1969).CrossRefGoogle Scholar
14. Cox, C.E., Lowe, B.G., and Sareen, R.A., IEEE Trans. Nucl. Sci. 35 (1), 8 (1988).CrossRefGoogle Scholar
15. Baertsch, R.D., IEEE Trans. Nuci. Sci. NS–18, 166 (1971).Google Scholar
16. Pehl, R.M., Cordi, R.C., and Goulding, F.S., IEEE Trans. Nuci. Sci. NS–19, 265 (1972).CrossRefGoogle Scholar
17. Maim, H.L., IEEE Trans. Nuci. Sci. NS–22 (1), 140 (1975).Google Scholar
18. Rossington, C.S., Walton, J.T., and Jaklevic, J.M., IEEE Trans. Nuci. Sci. 38, 239 (1991).Google Scholar
19. Rossington, C.S., Giauque, R.D., and Jaldevic, J.M., IEEE Trans. Nuci. Sci. 39, 570 (1992).CrossRefGoogle Scholar
20. Nashashibi, T. and White, G., IEEE Trans. Nut. Sci. 37 (2), 452 (1990).Google Scholar
21. Darken, L.S. and Jellison, G.E., (submitted for publication).Google Scholar
22. Darken, L.S., Phys. Rev. Aett. 69, 2839 (1992).Google Scholar
23. Actually, m* = mdC 3/2;/m† ½ where mdc is the density of states effective mass and m† is the mass required to give the right thermal velocity (3/2 kT = 1/2 m† <v>2). Equation (5) also approximates <v>2 = <v2>.42).+Equation+(5)+also+approximates+2+=+.4>Google Scholar
24. Darken, L.S. Jr., Trammell, R. C., Raudorf, T. W., Pehl, R. H., and Elliot, J.N., Nucl. Instr. Meth 171, 49 (1980).Google Scholar
25. Darken, L.S., Nucl. Instr. Meth Phys. Res. B (accepted for publication, 1993).Google Scholar
26. Darken, L.S., Trammell, R.C., Raudorf, T.W., and Pehl, R.H., IEEE Trans. Nut. Sci. NS–28, 572 (1981).Google Scholar
27. Fourches, N., Walter, G., and Bougoin, J.C., J. Appl. Phys. 69, 2033 (1991).Google Scholar