No CrossRef data available.
Article contents
Quasi-Two-Dimensional Smectic States of DNA Molecules Intercalated between Lipid Membranes in Multi-Lamellar Phases
Published online by Cambridge University Press: 15 February 2011
Abstract
We study fluctuations of DNA-cationic lipid complexes in their lamellar membrane phases with DNA intercalated between lipid membranes. We theoretically elucidate this novel state of matter by characterizing it as the very first realization of a decoupled (unregistered) phase of strongly fluctuating 2-d smectic manifolds weakly interacting across membranes. Due to couplings between adjacent 2-d smectic Lx, × Ly planes, the experimentally observed ordinary 2-d smectic behavior [Salditt et al., Phys. Rev. Lett. 79, 2582 (1997)] of DNA in-plane undulations, with 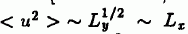 , must cross over, at the longest scales, to a novel fluctuation behavior, with < u2 > ˜ (logLy )2 ˜ (logLx.)2.
, must cross over, at the longest scales, to a novel fluctuation behavior, with < u2 > ˜ (logLy )2 ˜ (logLx.)2.
- Type
- Research Article
- Information
- Copyright
- Copyright © Materials Research Society 1998


