Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-26T03:10:53.836Z Has data issue: false hasContentIssue false
  • English
  • Français

Convolution Dirichlet Series and a Kronecker Limit Formula for Second-Order Eisenstein Series

Published online by Cambridge University Press:  11 January 2016

Jay Jorgenson  and
Cormac O’Sullivan
Jay Jorgenson
Affiliation:
Department of Mathematics, City College of New York, Convent Avenue at 138th Street New York, NY 10031, U.S.A.jjorgenson@mindspring.com
Cormac O’Sullivan
Affiliation:
Department of Mathematics, Bronx Community College, University Avenue and West 181st Street Bronx, NY 10453, U.S.A.cormac12@juno.com
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this article we derive analytic and Fourier aspects of a Kronecker limit formula for second-order Eisenstein series. Let Γ be any Fuchsian group of the first kind which acts on the hyperbolic upper half-space H such that the quotient Γ\H has finite volume yet is non-compact. Associated to each cusp of Γ\H, there is a classically studied first-order non-holomorphic Eisenstein series E(s, z) which is defined by a generalized Dirichlet series that converges for Re(s) > 1. The Eisenstein series E(s, z) admits a meromorphic continuation with a simple pole at s = 1. Classically, Kronecker’s limit formula is the study of the constant term 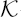1 (z) in the Laurent expansion of E(s, z) at s = 1. A number of authors recently have studied what is known as the second-order Eisenstein series E*(s, z), which is formed by twisting the Dirichlet series that defines the series E(s, z) by periods of a given cusp form f. In the work we present here, we study an analogue of Kronecker’s limit formula in the setting of the second-order Eisenstein series E* (s, z), meaning we determine the constant term 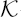2(z) in the Laurent expansion of E*(s, z) at its first pole, which is also at s = 1. To begin our investigation, we prove a bound for the Fourier coefficients associated to the first-order Kronecker limit function 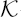1. We then define two families of convolution Dirichlet series, denoted by 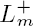and 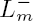with m ∈ ℕ, which are formed by using the Fourier coefficients of 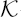1 and the weight two cusp form f. We prove that for all m, 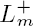and 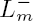admit a meromorphic continuation and are holomorphic at s = 1. Turning our attention to the second-order Kronecker limit function 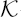2, we first express 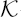2 as a solution to various differential equations. Then we obtain its complete Fourier expansion in terms of the cusp form f, the Fourier coefficients of the first-order Kronecker limit function 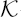1, and special values 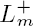(1) and 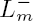(1) of the convolution Dirichlet series. Finally, we prove a bound for the special values 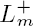(1) and 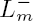(1) which then implies a bound for the Fourier coefficients of 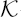2. Our analysis leads to certain natural questions concerning the holomorphic projection operator, and we conclude this paper by examining certain numerical examples and posing questions for future study.

Keywords

11F2011F3011F6711M41
Type
Research Article
Information
Nagoya Mathematical Journal , Volume 179 , 2005 , pp. 47 - 102
Copyright
Copyright © Editorial Board of Nagoya Mathematical Journal 2005

References

[As] Asai, T., On a certain function analogous to log η(z), Nagoya Math. J., 40 (1970), 193–211.CrossRefGoogle Scholar
[B-C-Z] Berndt, B., Chan, H. and Zhang, L. C., Ramanujan’s class invariants with applications to the values of q-continued fractions and theta functions, Special functions, q-series and related topics (Toronto, ON, 1995), Fields Inst. Commun., vol. 14, Amer. Math. Soc., Providence, R.I. (1997), pp. 37–53.Google Scholar
[Bu] Bump, D., Automorphic forms and representations, Cambridge University Press, Cambridge, 1997.CrossRefGoogle Scholar
[C-D-O’S] Chinta, G., Diamantis, N. and O’Sullivan, C., Second Order Modular Forms, Acta Arith., 103 (2002), 209–223.CrossRefGoogle Scholar
[C-O’S] Chinta, G. and O’Sullivan, C., Non-holomorphic Poincaré series constructed from derivatives and antiderivatives of cusp forms and bounds on period polynomials, to appear.Google Scholar
[C-I] Conrey, J. B. and Iwaniec, H., The cubic moment of central values of automorphic L-functions, Ann. of Math., 151 (2001), 1175–1216.CrossRefGoogle Scholar
[C-P] Chapman, R. and der Poorten, A. J. van, Binary quadratic forms and the eta function, Number Theory for the Millennium I, A. K. Peters (2002), pp. 215–227.Google Scholar
[E-G-M] Elstrodt, J., Grunewald, F. and Mennicke, J., Eisenstein series on three dimensional hyperbolic spaces and imaginary quadratic fields, Reine J. Math Angew., 360 (1985), 160–213.Google Scholar
[G-O’S] Goldfeld, D. and O’Sullivan, C., Estimating additive character sums for Fuchsian groups, Ramanujan J., 7 (2003), 241–267.CrossRefGoogle Scholar
[Gd] Goldfeld, D., The distribution of modular symbols, Number theory in progress, Vol. 2 (Zakopane - Kościelisko, 1997), de Gruyter, Berlin (1999), pp. 849–865.Google Scholar
[Gd2] Goldfeld, D., Modular forms, elliptic curves, and the ABC-conjecture, A panorama in number theory: The view from Baker’s garden. Based on a conference in honor of Alan Baker’s 60th birthday, Cambridge University Press, Cambridge (2002), pp. 128–147.CrossRefGoogle Scholar
[Gn] Goldstein, L. J., Dedekind sums for a Fuchsian group. I, Nagoya Math. J., 50 (1973), 21–47.CrossRefGoogle Scholar
[Iw1] Iwaniec, H., Spectral methods of automorphic forms, 2nd ed., Graduate studies in mathematics, vol. 53, Amer. Math. Soc., 2002.Google Scholar
[Iw2] Iwaniec, H., Topics in classical automorphic forms, Graduate studies in mathematics, vol. 17, Amer. Math. Soc., 1997.Google Scholar
[Iw3] Iwaniec, H., Fourier coefficients of modular forms and Kloosterman sums, unpublished lecture notes, 1987.Google Scholar
[J-L] Jorgenson, J. and Lang, S., Hilbert-Asai Eisenstein series, regularized products, and heat kernels, Nagoya Math. J., 153 (1999), 155–188.CrossRefGoogle Scholar
[Kub] Kubota, T., Elementary theory of Eisenstein series, Halsted Press (John Wiley and Sons), New York-London-Sydney, 1973.Google Scholar
[K-Z] Kleban, P. and Zagier, D., Crossing probabilities and modular forms, J. Stat. Phys., 113 (2003), 431–454.CrossRefGoogle Scholar
[La] Lang, S., Elliptic functions, 2nd ed., Springer-Verlag, 1987.CrossRefGoogle Scholar
[M-M] Manin, Y. and Marcolli, M., Continued fractions, modular symbols, and noncommutative geometry, Selecta Math. (N.S.), 8 (2002), 475–521.CrossRefGoogle Scholar
[Ne] Neunhöffer, H., Über die analytische Fortsetzung von Poincaréreihen, Sitzungsberichte Heidelberger Akad. Wiss., 2 (1973), 33–90.Google Scholar
[O’S1] O’Sullivan, C., Properties of Eisenstein series formed with modular symbols, J. Reine Angew. Math., 518 (2000), 163–186.Google Scholar
[O’S2] O’Sullivan, C., Identities from the holomorphic projection of modular forms, Number Theory for the Millennium III, A. K. Peters (2002), pp. 87–106.Google Scholar
[P-R] Petridis, Y. and Risager, M. S., Modular symbols have a normal distribution, Geom. Funct. Anal., 14 (2004), 1013–1043.CrossRefGoogle Scholar
[P-W] der Poorten, A. J. van and Williams, K. S., Values of the Dedekind eta function at quadratic irrationalities, Canad. J. Math., 51 (1999), 176–224.CrossRefGoogle Scholar
[Pe] Petridis, Y. N., Spectral deformations and Eisenstein series associated with modular symbols, Int. Math. Res. Not., 19 (2002), 991–1006.CrossRefGoogle Scholar
[Ra] Ramanathan, K. G., On some theorems stated by Ramanujan, Number Theory and related topics (Bombay 1988), Tata Inst. Fund. Res. Stud. Math., vol. 12, Tata Inst. Fund. Res., Bombay (1989), pp. 151–160.Google Scholar
[R-S] Ray, D. and Singer, I., Analytic torsion for complex manifolds, Ann. Math., 98 (1973), 154–177.CrossRefGoogle Scholar
[Ri] Risager, M. S., Ph.D. Thesis, University of Aarhus, 2003.Google Scholar
[Se] Selberg, A., On the estimation of Fourier coefficients of modular forms, Number Theory, Proc. Symposia in Pure Math., vol. 8, AMS Providence (1965), pp. 1–15.CrossRefGoogle Scholar
[Ser] Serre, J.-P., A Course in Arithmetic, Springer-Verlag, Berlin, 1973.CrossRefGoogle Scholar
[Sh] Shimura, G., Introduction to the Arithmetic Theory of Automorphic Functions, Princeton Univ. Press, Princeton, NJ, 1971.Google Scholar
[Si] Siegel, C. L., Lectures on Advanced Analytic Number Theory, Tata Institute, Bombay, 1961.Google Scholar
[Te] Terras, A., Harmonic Analysis on Symmetric Spaces and Applications I, Springer-Verlag, Berlin, 1985.CrossRefGoogle Scholar
[Za1] Zagier, D., Introduction to modular forms, From number theory to physics (Les Houches, 1989), Springer, Berlin (1992), pp. 238–291.Google Scholar
[Za2] Zagier, D., A Kronecker limit formula for real quadratic fields, Math. Ann., 213 (1975), 153–184.CrossRefGoogle Scholar