Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-26T18:53:01.466Z Has data issue: false hasContentIssue false
  • English
  • Français

LIBERATION, FREE MUTUAL INFORMATION AND ORBITAL FREE ENTROPY

Part of: Communication, information Selfadjoint operator algebras

Published online by Cambridge University Press:  14 September 2018

TAREK HAMDI
TAREK HAMDI*
Affiliation:
College of Business Administration, Qassim University, Buraydah, Saudi Arabia Laboratoire d’Analyse Mathématiques et applications LR11ES11, Université de Tunis El-Manar, Tunisie email tarek.hamdi@mail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we perform a detailed spectral study of the liberation process associated with two symmetries of arbitrary ranks: 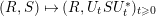$(R,S)\mapsto (R,U_{t}SU_{t}^{\ast })_{t\geqslant 0}$, where $(U_{t})_{t\geqslant 0}$ is a free unitary Brownian motion freely independent from 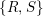$\{R,S\}$. Our main tool is free stochastic calculus which allows to derive a partial differential equation (PDE) for the Herglotz transform of the unitary process defined by 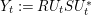$Y_{t}:=RU_{t}SU_{t}^{\ast }$. It turns out that this is exactly the PDE governing the flow of an analytic function transform of the spectral measure of the operator 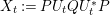$X_{t}:=PU_{t}QU_{t}^{\ast }P$ where 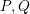$P,Q$ are the orthogonal projections associated to 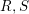$R,S$. Next, we relate the two spectral measures of 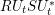$RU_{t}SU_{t}^{\ast }$ and of 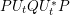$PU_{t}QU_{t}^{\ast }P$ via their moment sequences and use this relationship to develop a theory of subordination for the boundary values of the Herglotz transform. In particular, we explicitly compute the subordinate function and extend its inverse continuously to the unit circle. As an application, we prove the identity 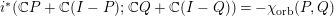$i^{\ast }(\mathbb{C}P+\mathbb{C}(I-P);\mathbb{C}Q+\mathbb{C}(I-Q))=-\unicode[STIX]{x1D712}_{\text{orb}}(P,Q)$.

MSC classification

Primary: 46L54: Free probability and free operator algebras
Secondary: 94A17: Measures of information, entropy
Type
Article
Information
Nagoya Mathematical Journal , Volume 239 , September 2020 , pp. 205 - 231
Check for updates
Copyright
© 2018 Foundation Nagoya Mathematical Journal

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Belinschi, S. T. and Bercovici, H., Partially defined semigroups relative to multiplicative free convolution, Int. Math. Res. Not. IMRN 2 (2005), 65–101.10.1155/IMRN.2005.65Google Scholar
Biane, P., Free Brownian Motion, Free Stochastic Calculus and Random Matrices, Fields Inst. Commun. 12, Amer. Math. Soc., Providence, RI, 1997, 1–19.Google Scholar
Cima, J., Matheson, A. L. and Ross, W. T., The Cauchy Transform, Mathematical Surveys and Monographs 125, Amer. Math. Soc., Providence, RI, 2006.10.1090/surv/125Google Scholar
Collins, B. and Kemp, T., Liberation of projections, J. Funct. Anal. 266 (2014), 1988–2052.10.1016/j.jfa.2013.10.034Google Scholar
Conway, J. B., Functions of One Complex Variable II, Graduate Texts in Mathematics, Springer-Verlag, New York, 1995.10.1007/978-1-4612-0817-4Google Scholar
Demni, N. and Hamdi, T., Inverse of the flow and moments of the free Jacobi process associated with one projection, Random Matrices: Theory Appl. (2) 07 (2018), 1850001.10.1142/S2010326318500016Google Scholar
Demni, N., Hamdi, T. and Hmidi, T., The spectral distribution of the free Jacobi process, Indiana Univ. Math. J. 61(3) (2012), 1351–1368.10.1512/iumj.2012.61.5034Google Scholar
Demni, N. and Hmidi, T., Spectral distribution of the free Jacobi process associated with one projection, Colloq. Math. 137(2) (2014), 271–296.10.4064/cm137-2-11Google Scholar
Duren, P. L., Theory of H p Spaces, Dover, Mineola, New York, 2000.Google Scholar
Dykema, K. J., Nica, A. and Voiculescu, D. V., Free Random Variables, CRM Monograph Series 1, American Mathematical Society, 1992.Google Scholar
Garnett, J. B., Bounded Analytic Functions, Pure and Applied Mathematics 96, Academic Press, New York, 1981.Google Scholar
Hiai, F., Miyamoto, T. and Ueda, Y., Orbital approach to microstate free entropy, Internat. J. Math. 20 (2009), 227–273.10.1142/S0129167X09005261Google Scholar
Hiai, F. and Petz, D., Large deviations for functions of two random projection matrices, Acta Sci. Math. (Szeged) 72 (2006), 581–609.Google Scholar
Hiai, F. and Ueda, Y., A log-Sobolev type inequality for free entropy of two projections, Ann. Inst. Henri Poincaré Probab. Stat. 45 (2009), 239–249.10.1214/08-AIHP164Google Scholar
Izumi, M. and Ueda, Y., Remarks on free mutual information and orbital free entropy, Nagoya Math. J 220 (2015), 45–66.10.1215/00277630-3335530Google Scholar
Lawler, G. F., Conformally Invariant Processes in the Plane, Mathematical Surveys and Monographs 114, Americal Mathematical Society, Providence, RI, 2005.Google Scholar
Nica, A. and Speicher, R., Lectures on the Combinatorics of Free Probability, Lecture Note Series 335, London Mathematical Society, Cambridge University Press, 2006.10.1017/CBO9780511735127Google Scholar
Ueda, Y., Orbital free entropy, revisited, Indiana Univ. Math. J. 63 (2014), 551–577.10.1512/iumj.2014.63.5220Google Scholar
Voiculescu, D. V., The analogues of entropy and of Fisher’s information measure in free probability theory. VI. Liberation and mutual free information, Adv. Math. 146(2) (1999), 101–166.10.1006/aima.1998.1819Google Scholar