No CrossRef data available.
Article contents
On Herstein’s Theorem Concerning Three Fields
Published online by Cambridge University Press: 22 January 2016
Extract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Let L > K ≧ Φ, L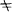 K, be three fields such that: (1) L/K is not purely inseparable, and (2) L/Φ is transcendental. Then Herstein’s theorem [2] asserts the existence of u ∈ L such that f(u) ∉ K for every non-constant polynomial f(X) ∈ Φ[X].
K, be three fields such that: (1) L/K is not purely inseparable, and (2) L/Φ is transcendental. Then Herstein’s theorem [2] asserts the existence of u ∈ L such that f(u) ∉ K for every non-constant polynomial f(X) ∈ Φ[X].
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Editorial Board of Nagoya Mathematical Journal 1961
References
[1]
Faith, Carl, A structure theory for semialgebraic extensions of division algebras, Journal für die reine und angewandte Mathematik, (1961).Google Scholar
[2]
Herstein, I. N., A theorem concerning three fields, Canadian Journal of Mathematics, vol. 7 (1955), 202–203.Google Scholar
[3]
Waerden, B. L. van der, Algebra I, Vierte Auflage, Berlin-Göttingen-Heidelberg, 1955.Google Scholar
[4]
Zariski, O., Interprétation algébrico-géométriques du quatorzième problème de Hilbert, Bull. Sci. Math. vol. 78 (1954), 155–168.Google Scholar

