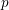Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-11T07:31:04.919Z Has data issue: false hasContentIssue false
  • English
  • Français

ON THE GALOIS STRUCTURE OF ARITHMETIC COHOMOLOGY I: COMPACTLY SUPPORTED $p$-ADIC COHOMOLOGY

Part of: Representation theory of groups Algebraic number theory: global fields

Published online by Cambridge University Press:  16 November 2018

DAVID BURNS
DAVID BURNS*
Affiliation:
King’s College London, Department of Mathematics, London WC2R 2LS, UK email david.burns@kcl.ac.uk
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate the Galois structures of 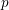$p$-adic cohomology groups of general 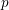$p$-adic representations over finite extensions of number fields. We show, in particular, that as the field extensions vary over natural families the Galois modules formed by these cohomology groups always decompose as the direct sum of a projective module and a complementary module of bounded 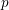$p$-rank. We use this result to derive new (upper and lower) bounds on the changes in ranks of Selmer groups over extensions of number fields and descriptions of the explicit Galois structures of natural arithmetic modules.

MSC classification

Primary: 11R34: Galois cohomology 11R23: Iwasawa theory 20C11: $p$-adic representations of finite groups
Type
Article
Information
Nagoya Mathematical Journal , Volume 239 , September 2020 , pp. 294 - 321
Check for updates
Copyright
© 2018 Foundation Nagoya Mathematical Journal

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Atiyah, M. F. and Wall, C. T. C., “Cohomology of groups”, in Algebraic Number Theory, (eds. Cassels, J. W. S. and Fröhlich, A.) Academic Press, London, 1967, 94–115.Google Scholar
Bloch, S. and Kato, K., “L-functions and Tamagawa numbers of motives”, in The Grothendieck Festschrift, Vol. I, Progress in Mathematics 86, Birkhäuser, 1990, 333–400.Google Scholar
Burns, D., On the Galois structure of arithmetic cohomology III: Selmer groups of critical motives, Kyoto J. Math. 58 (2018), 671–693.10.1215/21562261-2017-0034Google Scholar
Burns, D. and Flach, M., Motivic L-functions and Galois module structures, Math. Ann. 305 (1996), 65–102.10.1007/BF01444212Google Scholar
Burns, D. and Flach, M., Equivariant Tamagawa numbers for motives with (non-commutative) coefficients, Doc. Math. 6 (2001), 501–570.Google Scholar
Burns, D. and Kumon, A., On the Galois structure of arithmetic cohomology II: ray class groups, J. Math. Soc. Japan 70 (2018), 481–517.10.2969/jmsj/07027321Google Scholar
Burns, D., Macias Castillo, D. and Wuthrich, C., On the Galois structure of Selmer groups, Int. Math. Res. Not. 2015 (2015), 11909–11933.Google Scholar
Curtis, C. W. and Reiner, I., Methods of Representation Theory, Vol. I, John Wiley, New York, 1987.Google Scholar
Deligne, P., Seminaire de Geometrie Algebrique du Bois-Marie, SGA 4 1/2, Lecture Note Math. 569, Springer, New York, 1977.Google Scholar
Diederichsen, F. E., Über die Ausreduktion ganzzahliger Gruppendarstellungen bei arithmetischer Äquivalenz, Abh. Math. Sem. Univ. Hamburg 14 (1940), 357–412.Google Scholar
Flach, M., Euler characteristics in relative K-groups, Bull. Lond. Math. Soc. 32 (2000), 272–284.10.1112/S0024609300006950Google Scholar
Heller, A. and Reiner, I., Representations of cyclic groups in rings of integers. I, Ann. of Math. (2) 76 (1962), 73–92.10.2307/1970266Google Scholar
Heller, A. and Reiner, I., Representations of cyclic groups in rings of integers. II, Ann. of Math. (2) 77 (1963), 318–328.10.2307/1970218Google Scholar
Iwasawa, K., “On the 𝜇-invariants of ℤℓ-extensions”, in Number Theory, Algebraic Geometry and Commutative Algebra, Kinokuniya, Tokyo, 1973, 1–11.Google Scholar
Khare, C. and Wintenberger, J.-P., Ramification in Iwasawa theory and splitting conjectures, Int. Math. Res. Not. 2014 (2014), 194–223.10.1093/imrn/rns217Google Scholar
Milne, J. S., Étale Cohomology, Princeton University Press, Princeton, 1980.Google Scholar
Neukirch, J., On solvable extensions of number fields, Invent. Math. 53 (1979), 135–164.10.1007/BF01390030Google Scholar
Rzedowski-Calderón, M., Villa Salvador, G. D. and Madan, M. L., Galois module structure of rings of integers, Math. Z. 204 (1990), 401–424.10.1007/BF02570883Google Scholar
Verdier, J. L., Des Catégories Dérivées des Catégories Abéliennes, Asterisque 239, Société Mathématique de France, Montrouge, 1996.Google Scholar
Washington, L. C., Introduction to Cyclotomic Fields, Graduate Texts in Mathematics 83, Springer, Berlin, 1982.10.1007/978-1-4684-0133-2Google Scholar
Weibel, C., The norm residue isomorphism theorem, J. Topol. 2 (2009), 346–372.10.1112/jtopol/jtp013Google Scholar