Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-10T17:37:43.115Z Has data issue: false hasContentIssue false
  • English
  • Français

SELMER GROUPS OF ELLIPTIC CURVES OVER THE 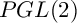$PGL(2)$ EXTENSION

Part of: Arithmetic algebraic geometry Algebraic number theory: global fields

Published online by Cambridge University Press:  30 May 2022

JISHNU RAY Open the ORCID record for JISHNU RAY [Opens in a new window]  and
R. SUJATHA Open the ORCID record for R. SUJATHA [Opens in a new window]
JISHNU RAY
Affiliation:
Institute for Advancing Intelligence TCG Centres for Research and Education in Science and Technology 1st Floor, Tower 1, Bengal Eco Intelligent Park (Techna Building) Block EM, Plot No 3, Sector V, Salt Lake Kolkata 700091, India jishnu.ray@tcgcrest.org, jishnuray1992@gmail.com
R. SUJATHA
Affiliation:
Department of Mathematics The University of British Columbia Room 121, 1984 Mathematics Road Vancouver, BC V6T 1Z2, Canada sujatha@math.ubc.ca
Get access Rights & Permissions [Opens in a new window]

Abstract

Iwasawa theory of elliptic curves over noncommutative 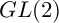$GL(2)$ extension has been a fruitful area of research. Over such a noncommutative p-adic Lie extension, there exists a structure theorem providing the structure of the dual Selmer groups for elliptic curves in terms of reflexive ideals in the Iwasawa algebra. The central object of this article is to study Iwasawa theory over the $PGL(2)$ extension and connect it with Iwasawa theory over the $GL(2)$ extension, deriving consequences to the structure theorem when the reflexive ideal is the augmentation ideal of the center. 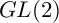We also show how the dual Selmer group over the $GL(2)$ extension being torsion is related with that of the 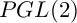$PGL(2)$ extension.

MSC classification

Primary: 11R23: Iwasawa theory
Secondary: 11G05: Elliptic curves over global fields 11R34: Galois cohomology
Type
Article
Information
Nagoya Mathematical Journal , Volume 248 , December 2022 , pp. 922 - 938
Check for updates
Copyright
© (2022) The Authors. The publishing rights in this article are licenced to Foundation Nagoya Mathematical Journal under an exclusive license

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

This work started with the Pacific Institute for the Mathematical Sciences and the Centre National de la Recherche Scientifique research funding received by Jishnu Ray. Later on, he also received funding from the Tata Institute of Fundamental Research and the Institute for Advancing Intelligence, The Chatterjee Group—Centres for Research and Education in Science and Technology in writing the revised versions. R. Sujatha gratefully acknowledges support from the Natural Sciences and Engineering Research Council of Canada Discovery grant 2019-03987.

References

Ardakov, K., Centres of skewfields and completely faithful Iwasawa modules , J. Inst. Math. Jussieu 7 (2008), 457–468.CrossRefGoogle Scholar
Backhausz, T. and Zábrádi, G., Algebraic functional equations and completely faithful Selmer groups , Int. J. Number Theory 11 (2015), 1233–1257.CrossRefGoogle Scholar
Bourbaki, N., Commutative Algebra. Chapters 1–7, Elem. Math. (Berlin), Springer, Berlin, 1998, translated from the French, reprint of the 1989 English translation.Google Scholar
Coates, J., “Fragments of the GL2 Iwasawa theory of elliptic curves without complex multiplication” in Arithmetic Theory of Elliptic Curves (Cetraro, 1997), Lecture Notes in Math. 1716, Springer, Berlin, 1999, 1–50.CrossRefGoogle Scholar
Coates, J., “Elliptic Curves — The Crossroads of Theory and Computation” In: Fieker, C., Kohel, D.R. (eds) Algorithmic Number Theory. ANTS 2002. Lecture Notes in Computer Science, vol 2369. Springer, Berlin, Heidelberg.Google Scholar
Coates, J., Fukaya, T., Kato, K., Sujatha, R., and Venjakob, O., The $G{L}_2$ main conjecture for elliptic curves without complex multiplication , Publ. Math. Inst. Hautes Études Sci. 101 (2005), 163–208.CrossRefGoogle Scholar
Coates, J. and Greenberg, R., Kummer theory for abelian varieties over local fields , Invent. Math. 124 (1996), 129–174.CrossRefGoogle Scholar
Coates, J. and Howson, S., Euler characteristics and elliptic curves , Proc. Natl. Acad. Sci. USA 94 (1997), 11115–11117, elliptic curves and modular forms (Washington, DC, 1996).CrossRefGoogle ScholarPubMed
Coates, J. and Howson, S., Euler characteristics and elliptic curves II , J. Math. Soc. Japan 53 (2001), 175–235.CrossRefGoogle Scholar
Coates, J., Schneider, P., and Sujatha, R., Modules over Iwasawa algebras , J. Inst. Math. Jussieu 2 (2003), 73–108.CrossRefGoogle Scholar
Coates, J. and Sujatha, R., Galois Cohomology of Elliptic Curves, 2nd ed., Narosa, New Delhi, 2010, for the Tata Institute of Fundamental Research, Mumbai.Google Scholar
Coates, J. and Sujatha, R., “On the MH (G)-conjecture” in Non-Abelian Fundamental Groups and Iwasawa Theory, London Math. Soc. Lecture Note Ser. 393, Cambridge Univ. Press, Cambridge, 2012, 132–161.Google Scholar
Cremona, J. E., Algorithms for Modular Elliptic Curves, 2nd ed., Cambridge Univ. Press, Cambridge, 1997.Google Scholar
Fisher, T., Descent calculations for the elliptic curves of conductor 11 , Proc. Lond. Math. Soc. 86 (2003), 583–606.CrossRefGoogle Scholar
Greenberg, R., “Iwasawa theory for elliptic curves” in Arithmetic Theory of Elliptic Curves (Cetraro, 1997), Lecture Notes in Math. 1716, Springer, Berlin, 1999, 51–144.CrossRefGoogle Scholar
Hachimori, Y. and Ochiai, T., Notes on non-commutative Iwasawa theory , Asian J. Math. 14 (2010), 11–18.CrossRefGoogle Scholar
Howson, S., Iwasawa theory of elliptic curves for $p$ -adic Lie extensions, Ph.D. dissertation, University of Cambridge, Cambridge, 1998.Google Scholar
Howson, S., Euler characteristics as invariants of Iwasawa modules , Proc. Lond. Math. Soc. 85 (2002), 634–658.CrossRefGoogle Scholar
Kato, K., p-adic Hodge theory and values of zeta functions of modular forms , Astérisque 295 (2004), 117–290, cohomologies $p$ -adiques et applications arithmétiques. III.Google Scholar
Mazur, B., Rational points of abelian varieties with values in towers of number fields , Invent. Math. 18 (1972), 183–266.CrossRefGoogle Scholar
Milne, J. S., Arithmetic duality theorems, Perspect. Math. 1, Academic Press, Boston, MA, 1986.Google Scholar
Neukirch, J., Schmidt, A., and Wingberg, K., Cohomology of Number Fields, 2nd ed., Grundlehren Math. Wiss. [Fund. Principles Math. Sci.] 323, Springer, Berlin, 2008.CrossRefGoogle Scholar
Ochi, Y. and Venjakob, O., On the structure of Selmer groups over p-adic Lie extensions , J. Algebraic Geom. 11 (2002), 547–580.CrossRefGoogle Scholar
Serre, J.-P., Propriétés galoisiennes des points d’ordre fini des courbes elliptiques , Invent. Math. 15 (1972), 259–331.CrossRefGoogle Scholar
Shekhar, S. and Sujatha, R., On the structure of Selmer groups of $\varLambda$ -adic deformations over $p$ -adic Lie extensions , Doc. Math. 17 (2012), 573–606.CrossRefGoogle Scholar
Venjakob, O., On the structure theory of the Iwasawa algebra of a $p$ -adic Lie group , J. Eur. Math. Soc. (JEMS) 4 (2002), 271–311.CrossRefGoogle Scholar
Zerbes, S., Selmer groups over $p$ -adic Lie extensions, Ph.D. dissertation, University of Cambridge, Cambridge, 2005.Google Scholar