Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-27T13:39:04.864Z Has data issue: false hasContentIssue false
  • English
  • Français

Robust a Simulation for Shallow Flows with Friction on Rough Topography

Published online by Cambridge University Press:  28 May 2015

Jian Deng ,
Ruo Li ,
Tao Sun  and
Shuonan Wu
Jian Deng*
Affiliation:
School of Mathematical Sciences, Peking University, Beijing 100871, China
Ruo Li*
Affiliation:
HEDPS & CAPT, LMAM & School of Mathematical Sciences, Peking University, Beijing 100871, China
Tao Sun*
Affiliation:
Reservoir Characterization Division ExxonMobil Upstream Research Company, P O. Box 2189 Houston, Texas 77252, USA
Shuonan Wu*
Affiliation:
School of Mathematical Sciences, Peking University, Beijing 100871, China
*
Corresponding author.Email address:lyricidyll3@gmail.com
Corresponding author.Email address:rli@math.pku.edu.cn
Corresponding author.Email address:tao.sun@exxonmobil.com
Corresponding author.Email address:wsnl987@gmail.com
Get access

Abstract

In this paper, we propose a robust finite volume scheme to numerically solve the shallow water equations on complex rough topography. The major difficulty of this problem is introduced by the stiff friction force term and the wet/dry interface tracking. An analytical integration method is presented for the friction force term to remove the stiffness. In the vicinity of wet/dry interface, the numerical stability can be attained by introducing an empirical parameter, the water depth tolerance, as extensively adopted in literatures. We propose a problem independent formulation for this parameter, which provides a stable scheme and preserves the overall truncation error of 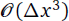. The method is applied to solve problems with complex rough topography, coupled with h-adaptive mesh techniques to demonstrate its robustness and efficiency.

Keywords

65M1065M1565N30Shallow waterfree interfaceManning force
Type
Research Article
Information
Numerical Mathematics: Theory, Methods and Applications , Volume 6 , Issue 2 , May 2013 , pp. 384 - 407
Copyright
Copyright © Global Science Press Limited 2013

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1]Molls, T., Zhao, G., and Molls, F., Friction slope in depth-averaged flow, Journal of Hydraulic Engineering, 124(1) (1998), pp. 81–85.CrossRefGoogle Scholar
[2]Bradford, S. F. and Sanders, B. F., Finite-volume model for shallow-water flooding of arbitrary topography, Journal of Hydraulic Engineering, 128(3) (2002), pp. 289–298.CrossRefGoogle Scholar
[3]Valiani, A. and Begnudelli, L., Divergence form for bed slope source term in shallow water equations, Journal of Hydraulic Engineering, 132(7) (2006), pp. 652–665.CrossRefGoogle Scholar
[4]Liang, Q. and Borthwick, A. G. L., Adaptive quadtree simulation of shallow flows with wet-dry fronts over complex topography, Computers and Fluids, 38(2) (2009), pp. 221–234.CrossRefGoogle Scholar
[5]Hu, K., Mingham, C. G., and Causon, D. M., Numerical simulation of wave overtopping of coastal structures using the non-linear shallow water equations, Coastal Engineering, 41(4) (2000), pp. 433–465.CrossRefGoogle Scholar
[6]Yoon, T. H. and Kang, S. K., Finite volume model for two-dimensional shallow water flows on unstructured grids, Journal of Hydraulic Engineering, 130(7) (2004), pp. 678–688.CrossRefGoogle Scholar
[7]Valiani, A., Caleffi, V., and Zanni, A., Case study: Malpasset dam-break simulation using a two-dimensional finite volume method, Journal of Hydraulic Engineering, 128(5) (2002), pp. 460–472.CrossRefGoogle Scholar
[8]Anastasiou, K. and Chan, C. T., Solution of the 2D shallow water equations using the finite volume method on unstructured triangular meshes, International Journal for Numerical Methods in Fluids, 24(11) (1997), pp. 1225–1245.3.0.CO;2-D>CrossRefGoogle Scholar
[9]Audusse, E., Bouchut, F., Bristeau, M. O., Klein, R., and Perthame, B., A fast and stable well-balanced scheme with hydrostatic reconstruction for shallow water flows, SIAM Journal on Scientific Computing, 25(6) (2004), pp. 2050–2065.CrossRefGoogle Scholar
[10]Kurganov, A. and Petrova, G., A second-order well-balanced positivity preserving central-upwind scheme for the Saint-Venant system, Communications in Mathematical Sciences, 5(1) (2007), pp. 133–160.CrossRefGoogle Scholar
[11]Castro, M. J., González-Vida, J. M., Pares, C., and Bellomo, N., Numerical treatment of wet/dry fronts in shallow flows with a modified Roe scheme, Mathematical Models and Methods in Applied Sciences, 16(6) (2006), pp. 897–932.CrossRefGoogle Scholar
[12]Ricchiuto, M. and Bollermann, A., Stabilized residual distribution for shallow water simulations, Journal of Computational Physics, 228(4) (2009), pp. 1071–1115.CrossRefGoogle Scholar
[13]Bollermann, A., Noelle, S., and Lukáčová-Medvid’ová, M., Finite volume evolution Galerkin methods for the shallow water equations with dry beds, Communications in Computational Physics, 10(2) (2011), pp. 371–404.CrossRefGoogle Scholar
[14]Gallardo, J. M., Pares, C., and Castro, M., On a well-balanced high-order finite volume scheme for shallow water equations with topography and dry areas, Journal of Computational Physics, 227(1) (2007), pp. 574–601.CrossRefGoogle Scholar
[15]Bradford, S. F. and Katopodes, N. D., Hydrodynamics of turbid underflows. I: Formulation and numerical analysis, Journal of Hydraulic Engineering, 125(10) (1999), pp. 1006–1015.CrossRefGoogle Scholar
[16]Brufau, P. and García-Navarro, P., Unsteady free surface flow simulation over complex topography with a multidimensional upwind technique, Journal of Computational Physics, 186(2) (2003), pp. 503–526.CrossRefGoogle Scholar
[17]Begnudelli, L. and Sanders, B. F., Unstructured grid finite-volume algorithm for shallow-water flow and scalar transport with wetting and drying, Journal of Hydraulic Engineering, 132(4) (2006), pp. 371–384.CrossRefGoogle Scholar
[18]Vreugdenhil, C. B., Numerical Methods for Shallow-Water Flow, Springer, 1994.CrossRefGoogle Scholar
[19]Tseng, M. H., Improved treatment of source terms in TVD scheme for shallow water equations, Advances in Water Resources, 27(6) (2004), pp. 617–629.CrossRefGoogle Scholar
[20]Strang, G., On the construction and comparison of difference schemes, SIAM Journal on Numerical Analysis, 5(3) (1968), pp. 506–517.CrossRefGoogle Scholar
[21]Albada, G. D. Van, Leer, B.Van, and Roberts, W. W. jr., A comparative study of computational methods in cosmic gas dynamics, Astronomy and Astrophysics, 108 (1982), pp. 76–84.Google Scholar
[22]Harten, A., Lax, P. D., and Leer, B. Van, On upstream differencing and Godunov-type schemes for hyperbolic conservation laws, SIAM Review, (1983), pp. 35–61.CrossRefGoogle Scholar
[23]Zhou, J. G., Causon, D. M., Mingham, C. G., and Ingram, D. M., The surface gradient method for the treatment of source terms in the shallow-water equations, Journal of Computational Physics, 168(1) (2001), pp. 1–25.CrossRefGoogle Scholar
[24]Abgrall, R., On essentially non-oscillatory schemes on unstructured meshes: analysis and implementation, NASA Contractor Report 191415 (1992).Google Scholar
[25]Sleigh, P. A., Gaskell, P. H., Berzins, M., and Wright, N. G., An unstructured finite-volume algorithm f or predicting flow in rivers and estuaries, Computers and Fluids, 27(4) (1998), pp. 479–508.CrossRefGoogle Scholar
[26]Leveque, R. J. and Oliger, J., Numerical methods based on additive splittings for hyperbolic partial differential equations, Mathematics of Computation, 40(162) (1983), pp. 469–497.CrossRefGoogle Scholar
[27]Li, R. and Liu, W. B., http://dsec.pku.edu.cn/~rli/.Google Scholar
[28]Li, R., On multi-mesh h-adaptive methods, Journal of Scientific Computing, 24(3) (2005), pp. 321–341.CrossRefGoogle Scholar
[29]Li, R., and Wu, S.-N., H-adaptive mesh method with double tolerance adaptive strategy for hyperbolic conservation laws, DOI: 10.1007/s10915-013-9692-1, 2013.CrossRefGoogle Scholar
[30]Thacker, W. C., Some exact solutions to the nonlinear shallow-water wave equations, Journal of Fluid Mechanics, 107(1) (1981), pp. 499–508.CrossRefGoogle Scholar
[31]Sampson, J., Easton, A., and Singh, M., Moving boundary shallow water flow above parabolic bottom topography, ANZIAM Journal, 47 (2006), pp. C373–C387.CrossRefGoogle Scholar
[32]Wang, Y., Liang, Q., Kesserwani, G., and Hall, J. W., A 2D shallow flow model for practical dam-break simulations, Journal of Hydraulic Research, 49(3) (2011), pp. 307–316.CrossRefGoogle Scholar
[33]Kawahara, M. and Umetsu, T., Finite element method for moving boundary problems in river flow, International Journal for Numerical Methods in Fluids, 6(6) (1986), pp. 365–386.CrossRefGoogle Scholar
[34]Brufau, P., Vázquez-Cendón, M. E., and García-Navarro, P., A numerical model for the flooding and drying of irregular domains, International Journal for Numerical Methods in Fluids, 39(3) (2002), pp. 247–275.CrossRefGoogle Scholar
[35]Hervouet, J.M., Hydrodynamics of Free Surface Flows: Modelling with the Finite Element Method, John Wiley & Sons Inc, 2007.CrossRefGoogle Scholar
[36]Hervouet, J. M. and Petitjean, A., Malpasset dam-break revisited with two-dimensional computations, Journal of Hydraulic Research, 37(6) (1999), pp. 777–788.CrossRefGoogle Scholar