Article contents
Stochastic Comparison of Some Wear Processes
Published online by Cambridge University Press: 27 July 2009
Abstract
Let [NA(t), t≥0] and [NB(t), t≥0] be two counting processes with independent interarrivals Ai and Bi, respectively, i ∈ 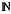 + ≡ [1,2,… ], and let [Xi, i ∈
+ ≡ [1,2,… ], and let [Xi, i ∈ 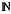 +] and [Yi, i ∈
+] and [Yi, i ∈ 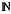 +] be two sequences of independent non-negative random variables. Consider the two compound processes [U(t), t ≥ 0] and [V(t), t ≥ 0] defined as U(t) =
+] be two sequences of independent non-negative random variables. Consider the two compound processes [U(t), t ≥ 0] and [V(t), t ≥ 0] defined as U(t) = 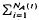 Xi, t ≥ 0, and V(t) =
Xi, t ≥ 0, and V(t) =  Yi, t ≥ 0. Then it is well known (and not hard to verify) that if the Ai's are independent of the Xi's, and if the Bi's are independent of the yi's, and if Ai ≥st Bi and Xi ≤st Yi, for all i ∈
Yi, t ≥ 0. Then it is well known (and not hard to verify) that if the Ai's are independent of the Xi's, and if the Bi's are independent of the yi's, and if Ai ≥st Bi and Xi ≤st Yi, for all i ∈ 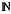 +, then [U(t), t ≥ 0] ≤st [V(t), t ≥ 0]. In this paper we provide conditions under which this stochastic order relation holds even if Ai ≤st Bi and/or Xi ≥st Yi for all i ∈
+, then [U(t), t ≥ 0] ≤st [V(t), t ≥ 0]. In this paper we provide conditions under which this stochastic order relation holds even if Ai ≤st Bi and/or Xi ≥st Yi for all i ∈ 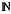 +. In fact, we derive the stochastic order relationships among processes that are much more general than the cumulative ones described above. For example, it is not necessarily assumed that the Ai's are independent of the Xi's or that the Bi's are independent of the Yi's. Examples that illustrate the applications of the theory are included.
+. In fact, we derive the stochastic order relationships among processes that are much more general than the cumulative ones described above. For example, it is not necessarily assumed that the Ai's are independent of the Xi's or that the Bi's are independent of the Yi's. Examples that illustrate the applications of the theory are included.
Information
- Type
- Research Article
- Information
- Probability in the Engineering and Informational Sciences , Volume 7 , Issue 3 , July 1993 , pp. 421 - 435
- Copyright
- Copyright © Cambridge University Press 1993
References
- 7
- Cited by

