Published online by Cambridge University Press: 05 November 2020
Let p be prime. We prove that, for n odd, the p-torsion part of πq(Sn) has cardinality at most $p^{2^{{1}/({p-1})(q-n+3-2p)}}$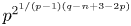 and hence has rank at most 21/(p−1)(q−n+3−2p). for p = 2, these results also hold for n even. The best bounds proven in the existing literature are $p^{2^{q-n+1}}$
and hence has rank at most 21/(p−1)(q−n+3−2p). for p = 2, these results also hold for n even. The best bounds proven in the existing literature are $p^{2^{q-n+1}}$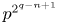 and 2q−n+1, respectively, both due to Hans–Werner Henn. The main point of our result is therefore that the bound grows more slowly for larger primes. As a corollary of work of Henn, we obtain a similar result for the homotopy groups of a broader class of spaces.
and 2q−n+1, respectively, both due to Hans–Werner Henn. The main point of our result is therefore that the bound grows more slowly for larger primes. As a corollary of work of Henn, we obtain a similar result for the homotopy groups of a broader class of spaces.