Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-10T16:54:38.622Z Has data issue: false hasContentIssue false
  • English
  • Français

Conditions for Sylow 2-subgroups of the Fixed Point Subgroup Implying Solubility

Part of: Representation theory of groups

Published online by Cambridge University Press:  25 September 2018

Antonio Beltrán  and
Changguo Shao
Antonio Beltrán
Affiliation:
Departamento de Matemáticas, Universidad Jaume I, 12071 Castellón, Spain (abeltran@mat.uji.es)
Changguo Shao
Affiliation:
School of Mathematical Science, University of Jinan, 250022 Shandong, China (shaoguozi@163.com)
Get access Rights & Permissions [Opens in a new window]

Abstract

Let A and G be finite groups and suppose that A acts via automorphisms on G with 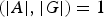$(|A|, |G|)=1$. We study how certain conditions on the Sylow 2-subgroups of the fixed point subgroup of the action 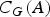$C_G(A)$ may imply the non-simplicity or solubility of G.

Keywords

finite groupsSylow subgroupscoprime actiongroup action on groups

MSC classification

Primary: 20D20: Sylow subgroups, Sylow properties, $pi$-groups, $pi$-structure
Secondary: 20D15: Nilpotent groups, $p$-groups
Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 62 , Issue 1 , February 2019 , pp. 211 - 220
Copyright
Copyright © Edinburgh Mathematical Society 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Beltrán, A., Invariant Sylow subgroups and solvability of finite groups, Arch. Math. 102(2) (2016), 101–106.Google Scholar
2Brauer, R. and Suzuki, M., On finite groups of even order whose 2-Sylow group is a quaternion group, Proc. Natl. Acad. Sci. USA 45 (1959), 1757–1759.Google Scholar
3Chen, Z. M. and Wang, Y. M., Minimal non-solvable groups with an acting group (in Chinese), Chin. Sci. Bull. 34(22) (1989), 1691–1693.Google Scholar
4Conway, J. H., Curtis, R. T., Norton, S. P., Parker, R. A. and Wilson, R. A. Atlas of finite groups (Oxford University Press, London, 1985).Google Scholar
5Gorenstein, D., Finite groups (Chelsea Pub. Co., New York, 1980).Google Scholar
6Kurzweil, H. and Stellmacher, B., The theory of finite groups. An introduction (Springer-Verlag, Berlin–Heidelberg–New York, 2004).Google Scholar
7Walter, J. H., The characterization of finite groups with abelian Sylow 2-subgroups, Ann. Math. 89 (1969), 405–514.Google Scholar
8Walter, G. and Gorenstein, J. H., On finite groups with dihedral Sylow 2-subgroups, Illinois J. Math. 6 (1962), 553–593.Google Scholar