Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Yang, Xiaoyan
and
Zhao, Jianlian
2013.
Gorenstein Flat and Cotorsion Dimensions of Unbounded Complexes.
Communications in Algebra,
Vol. 41,
Issue. 8,
p.
2978.
Yang, Gang
Liu, Zhongkui
and
Liang, Li
2013.
Model Structures on Categories of Complexes Over Ding-Chen Rings.
Communications in Algebra,
Vol. 41,
Issue. 1,
p.
50.
WEI, REN
and
LIU, ZHONGKUI
2014.
A QUILLEN MODEL STRUCTURE APPROACH TO HOMOLOGICAL DIMENSIONS OF COMPLEXES.
Journal of Algebra and Its Applications,
Vol. 13,
Issue. 03,
p.
1350106.
YANG, GANG
and
LIANG, LI
2014.
CARTAN–EILENBERG GORENSTEIN PROJECTIVE COMPLEXES.
Journal of Algebra and Its Applications,
Vol. 13,
Issue. 01,
p.
1350068.
Šťovíček, Jan
2014.
Derived equivalences induced by big cotilting modules.
Advances in Mathematics,
Vol. 263,
Issue. ,
p.
45.
Yang, Gang
and
Du, Rui-Juan
2015.
On Cotorsion Pairs of Chain Complexes.
Communications in Algebra,
Vol. 43,
Issue. 3,
p.
959.
Hu, Jiangsheng
Geng, Yuxian
Xie, Zhengwei
and
Zhang, Dongdong
2015.
GorensteinFP-Injective Dimension for Complexes.
Communications in Algebra,
Vol. 43,
Issue. 8,
p.
3515.
Gillespie, James
2016.
Gorenstein complexes and recollements from cotorsion pairs.
Advances in Mathematics,
Vol. 291,
Issue. ,
p.
859.
Hu, Jiangsheng
Geng, Yuxian
and
Jiang, Qinghua
2016.
Complete cohomology for complexes with finite Gorenstein AC-projective dimension.
Frontiers of Mathematics in China,
Vol. 11,
Issue. 4,
p.
901.
Hu, Jiangsheng
Liu, Haiyu
and
Geng, Yuxian
2016.
When every pure ideal is projective.
Journal of Algebra and Its Applications,
Vol. 15,
Issue. 02,
p.
1650030.
Hu, Jiangsheng
and
Ding, Nanqing
2016.
A model structure approach to the Tate–Vogel cohomology.
Journal of Pure and Applied Algebra,
Vol. 220,
Issue. 6,
p.
2240.
Gillespie, James
2016.
Hereditary abelian model categories.
Bulletin of the London Mathematical Society,
Vol. 48,
Issue. 6,
p.
895.
Odabaşı, Sinem
2016.
Cotorsion Pairs and Cartan–Eilenberg Categories.
Mediterranean Journal of Mathematics,
Vol. 13,
Issue. 4,
p.
1479.
Estrada, Sergio
Fu, Xianhui
and
Iacob, Alina
2017.
Totally acyclic complexes.
Journal of Algebra,
Vol. 470,
Issue. ,
p.
300.
Trlifaj, Jan
2017.
Groups, Modules, and Model Theory - Surveys and Recent Developments.
p.
191.
Di, Zhenxing
Zhang, Xiaoxiang
and
Chen, Jianlong
2017.
Gorenstein AC-projective dimension of unbounded complexes.
Communications in Algebra,
Vol. 45,
Issue. 7,
p.
2855.
Gillespie, James
2017.
On the homotopy category of AC-injective complexes.
Frontiers of Mathematics in China,
Vol. 12,
Issue. 1,
p.
97.
Yang, Xiaoyan
and
Chen, Wenjing
2017.
Relative homological dimensions and Tate cohomology of complexes with respect to cotorsion pairs.
Communications in Algebra,
Vol. 45,
Issue. 7,
p.
2875.
Gillespie, James
2017.
On Ding injective, Ding projective and Ding flat modules and complexes.
Rocky Mountain Journal of Mathematics,
Vol. 47,
Issue. 8,
Chen, Wenjing
Liu, Zhongkui
and
Yang, Xiaoyan
2018.
Compactly generated triangulated subcategories of homotopy categories induced by cotorsion pairs.
Journal of Algebra and Its Applications,
Vol. 17,
Issue. 09,
p.
1850180.


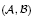 in the category of modules is complete and hereditary, then both of the induced cotorsion pairs in the category of complexes are complete. We also give a cofibrantly generated model structure that can be regarded as a generalization of the projective model structure.
in the category of modules is complete and hereditary, then both of the induced cotorsion pairs in the category of complexes are complete. We also give a cofibrantly generated model structure that can be regarded as a generalization of the projective model structure.