No CrossRef data available.
Article contents
A criterion for absolute Cesàro summability of negative order of a Fourier series
Published online by Cambridge University Press: 20 January 2009
Extract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Let f(x) be integrable L(0, 2π) and periodic with period 2π, and let ψ(t) be the conjugate function of 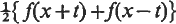 with respect to the variable t, where x is onsidered as an arbitrary constant. The following theorems are due to K. K. Chen (1), (2), pp. 111–124.
with respect to the variable t, where x is onsidered as an arbitrary constant. The following theorems are due to K. K. Chen (1), (2), pp. 111–124.
Information
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 14 , Issue 4 , December 1965 , pp. 311 - 319
- Copyright
- Copyright © Edinburgh Mathematical Society 1965
References
REFERENCES
(1) Chen, K. K.On the absolute Cesaro summability of negative order for a Fourier series at a given point. Amer. J. Math. 66 (1944), 299–312.CrossRefGoogle Scholar
(2) Chen, K. K.Summation of the Fourier Series of Orthogonal Functions (Peking, 1957).Google Scholar
(3) Chow, H. C.Note on the absolute Cesaro summability of power series. Proc. London Math. Soc. (2) 43 (1937), 484–489.Google Scholar
(4) Chow, H. C.The absolute summability of Fourier series. J. London Math. Soc. 17 (1942), 17–23.CrossRefGoogle Scholar
(5) Flett, T. M.On an extension of absolute summability and some theorems of Littlewood and Paley. Proc. London Math. Soc. (3) 7 (1957), 113–141.CrossRefGoogle Scholar
(6) Flett, T. M.On the absolute summability of a Fourier series and its con- jugate series. Proc. London Math. Soc. (3) 8 (1958), 257–311.Google Scholar
(7) vFLETT, T. M.Some more theorems concerning the absolute summability of Fourier series and power series. Proc. London Math. Soc. (3) 8 (1958), 359–387.Google Scholar
(8) Hardy, G. H. and Littlewood, J. E.A convergence criterion for Fourier series. Math. Zeitschrift, 28, (1928), 612–634.CrossRefGoogle Scholar
(9) Hardy, G. H. and Littlewood, J. E.Some properties of fractional integrals, I. Math. Zeitschrift, 27 (1928), 565–606.CrossRefGoogle Scholar
(10) Hyslop, J. M.On the absolute summability of Fourier series. Proc. London Math. Soc. (2) 43 (1937), 475–483.Google Scholar
(11) Kogbetliantz, E.Sur les series absolument sommables par la mdthode des moyennes arithméique. Bull, des Sci. Math. (2) 49 (1925), 234–256.Google Scholar
(12) Zygmund, A.Remarque sur la convergence absolue des series de Fourier. J. London Math. Soc. 3 (1928), 194–196.CrossRefGoogle Scholar

