Published online by Cambridge University Press: 20 January 2009
Indices of the free logarithmetic 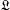 correspond to bifurcating root-trees (cf.(4)), to Evans' non-associative numbers (3) and to Etherington's partitive numbers (2). The free commutative logarithmetic
correspond to bifurcating root-trees (cf.(4)), to Evans' non-associative numbers (3) and to Etherington's partitive numbers (2). The free commutative logarithmetic 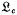 is the homomorph of f
is the homomorph of f 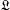 determined by the congruence relation P + Q ∼ Q + P. Formulæ for aδ and pα, i.e. the numbers of indices of
determined by the congruence relation P + Q ∼ Q + P. Formulæ for aδ and pα, i.e. the numbers of indices of 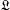 of a given potency* δ and the number of indices of a given altitude α respectively, were given by Etherington (1), who also gave corresponding formulæ for commutative indices of
of a given potency* δ and the number of indices of a given altitude α respectively, were given by Etherington (1), who also gave corresponding formulæ for commutative indices of 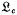 . Other enumeration formulæ are contained in (5).
. Other enumeration formulæ are contained in (5).