Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-26T05:53:32.024Z Has data issue: false hasContentIssue false
  • English
  • Français

A note on Taskinen's counterexamples on the problem of topologies of Grothendieck

Published online by Cambridge University Press:  20 January 2009

Jose Bonet  and
Antonio Galbis
Jose Bonet
Affiliation:
Departamento de Matemáticas, E.T.S. Arquitectura, Universidad Politécnica de Valencia, 46022 Valencia, Spain
Antonio Galbis
Affiliation:
Departamento de Análisis, Facultad de Matemáticas, Universidad de Valencia, 46071 Burjasot (Valencia), Spain
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

By the work of Taskinen (see [4, 5]), we know that there is a Fréchet space E such that Lb(E, l2) is not a (DF)-space. Moreover there is a Fréchet–Montel space F such that 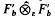is not (DF). In this second example, the duality theorem of Buchwalter (cf. [2, §45.3]) can be applied to obtain that 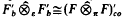and hence is a (gDF)-space (cf. [1, Ch. 12 or 3, Ch. 8]). The (gDF)-spaces were introduced by several authors to extend the (DF)-spaces of Grothendieck and to provide an adequate frame to consider strict topologies.

Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 32 , Issue 2 , June 1989 , pp. 281 - 283
Copyright
Copyright © Edinburgh Mathematical Society 1989

References

REFERENCES

1.Jarchow, H., Locally convex spaces (B. G. Teubner, Stuttgart, 1981).CrossRefGoogle Scholar
2.Kothe, G., Topological vector spaces II (Springer-Verlag, 1969).Google Scholar
3.Carreras, P. Perez and Bonet, J., Barrelled locally convex spaces (North-Holland Math. Studies, 131, 1987).CrossRefGoogle Scholar
4.Taskinen, J., Counterexamples to “Probleme des topologies” of Grothendieck, Ann. Acad. Sci. Fenn. Ser. A, I. Math. Dissertationes 63 (1986).Google Scholar
5.Taskinen, J., The projective tensor product of Fréchet-Montel spaces, Studia Math. 91 (1988), 17–30.CrossRefGoogle Scholar