No CrossRef data available.
Article contents
The Point Value Maximization Problem for Positive Definite Functions Supported in a Given Subset of a Locally Compact Group
Published online by Cambridge University Press: 24 January 2018
Abstract
The century-old extremal problem, solved by Carathéodory and Fejér, concerns a non-negative trigonometric polynomial 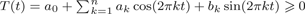 $T(t) = a_0 + \sum\nolimits_{k = 1}^n {a_k} \cos (2\pi kt) + b_k\sin (2\pi kt){\ge}0$ , normalized by a 0=1, where the quantity to be maximized is the coefficient a 1 of cos (2π t). Carathéodory and Fejér found that for any given degree n, the maximum is 2 cos(π/n+2). In the complex exponential form, the coefficient sequence (c k ) ⊂ ℂ will be supported in [−n, n] and normalized by c 0=1. Reformulating, non-negativity of T translates to positive definiteness of the sequence (c k ), and the extremal problem becomes a maximization problem for the value at 1 of a normalized positive definite function c: ℤ → ℂ, supported in [−n, n]. Boas and Kac, Arestov, Berdysheva and Berens, Kolountzakis and Révész and, recently, Krenedits and Révész investigated the problem in increasing generality, reaching analogous results for all locally compact abelian groups. We prove an extension to all the known results in not necessarily commutative locally compact groups.
$T(t) = a_0 + \sum\nolimits_{k = 1}^n {a_k} \cos (2\pi kt) + b_k\sin (2\pi kt){\ge}0$ , normalized by a 0=1, where the quantity to be maximized is the coefficient a 1 of cos (2π t). Carathéodory and Fejér found that for any given degree n, the maximum is 2 cos(π/n+2). In the complex exponential form, the coefficient sequence (c k ) ⊂ ℂ will be supported in [−n, n] and normalized by c 0=1. Reformulating, non-negativity of T translates to positive definiteness of the sequence (c k ), and the extremal problem becomes a maximization problem for the value at 1 of a normalized positive definite function c: ℤ → ℂ, supported in [−n, n]. Boas and Kac, Arestov, Berdysheva and Berens, Kolountzakis and Révész and, recently, Krenedits and Révész investigated the problem in increasing generality, reaching analogous results for all locally compact abelian groups. We prove an extension to all the known results in not necessarily commutative locally compact groups.
Keywords
Information
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 61 , Issue 1 , February 2018 , pp. 179 - 200
- Copyright
- Copyright © Edinburgh Mathematical Society 2018

