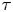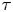Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Wang, Qi
2022.
On τ-tilting finiteness of the Schur algebra.
Journal of Pure and Applied Algebra,
Vol. 226,
Issue. 1,
p.
106818.
Ariki, Susumu
Lyle, Sinéad
and
Speyer, Liron
2023.
Schurian‐finiteness of blocks of type A$A$ Hecke algebras.
Journal of the London Mathematical Society,
Vol. 108,
Issue. 6,
p.
2333.
Kozakai, Yuta
2023.
On $$\tau $$-tilting finiteness of block algebras of direct products of finite groups.
Bulletin of the Iranian Mathematical Society,
Vol. 49,
Issue. 3,
AIHARA, TAKUMA
and
HONMA, TAKAHIRO
2024.
WHEN IS THE SILTING-DISCRETENESS INHERITED?.
Nagoya Mathematical Journal,
Vol. 256,
Issue. ,
p.
905.
Miyamoto, Kengo
and
Wang, Qi
2024.
On $\tau$-tilting Finiteness of Symmetric Algebras of Polynomial Growth.
Taiwanese Journal of Mathematics,
Vol. 28,
Issue. 6,
Iyama, Osamu
and
Kimura, Yuta
2024.
Classifying subcategories of modules over Noetherian algebras.
Advances in Mathematics,
Vol. 446,
Issue. ,
p.
109631.
Chen, Xiao-Wu
Li, Zhi-Wei
Zhang, Xiaojin
and
Zhao, Zhibing
2024.
A non-vanishing result on the singularity category.
Proceedings of the American Mathematical Society,
Asai, Sota
and
Iyama, Osamu
2024.
Semistable torsion classes and canonical decompositions in Grothendieck groups.
Proceedings of the London Mathematical Society,
Vol. 129,
Issue. 5,
Hiramae, Naoya
and
Kozakai, Yuta
2025.
𝜏-Tilting finiteness of group algebras of semidirect products of abelian 𝑝-groups and abelian 𝑝′-groups.
Journal of Group Theory,