No CrossRef data available.
Published online by Cambridge University Press: 30 April 2021
We prove that solutions to the quintic semilinear wave equation with variable coefficients in ${{\mathbb {R}}}^{1+3}$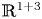 scatter to a solution to the corresponding linear wave equation. The coefficients are small and decay as $|x|\to \infty$
scatter to a solution to the corresponding linear wave equation. The coefficients are small and decay as $|x|\to \infty$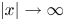 , but are allowed to be time dependent. The proof uses local energy decay estimates to establish the decay of the $L^{6}$
, but are allowed to be time dependent. The proof uses local energy decay estimates to establish the decay of the $L^{6}$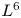 norm of the solution as $t\to \infty$
norm of the solution as $t\to \infty$ .
.