No CrossRef data available.
Published online by Cambridge University Press: 20 January 2009
Let A be a complex unital Banach algebra. An element u∈A is a norm unitary if
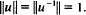
(For the algebra of all bounded operators on a Banach space, the norm unitaries arethe invertible isometries.) Given a norm unitary u∈A, we have Sp(u)⊃Γ, where Sp(u) denotes the spectrum of u and Γ denotes the unit circle in C. If Sp(u)≠Γ we may suppose, by replacing eiθu, that 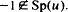 . Then there exists h ∈ A such that
. Then there exists h ∈ A such that