Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Petrich, Mario
1994.
Congruence networks for completely simple semigroups.
Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics,
Vol. 56,
Issue. 2,
p.
243.
Feng, Ying-Ying
and
Wang, Li-Min
2010.
Congruence Networks on Regular Semigroups Associated with 𝒱-Relation.
Communications in Algebra,
Vol. 38,
Issue. 4,
p.
1449.

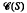 be its congruence lattice. For ρ ∈
be its congruence lattice. For ρ ∈ 