Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Bentley, H. L.
1983.
HOMOLOGY AND COHOMOLOGY FOR MEROTOPIC AND NEARNESS SPACES.
Quaestiones Mathematicae,
Vol. 6,
Issue. 1-3,
p.
27.


 be a covering of a topological space
be a covering of a topological space 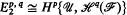 and
and  for some filtration of the graded group
for some filtration of the graded group 