No CrossRef data available.
Article contents
Supremum and infimum of subharmonic functions of order between 1 and 2
Part of:
Two-dimensional theory
Published online by Cambridge University Press: 08 April 2011
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
For functions u, subharmonic in the plane, let

and let N(r,u) be the integrated counting function. Suppose that  is a non-negative non-decreasing convex function of log r for which
is a non-negative non-decreasing convex function of log r for which  for all small r and
for all small r and  , where 1 < ρ < 2, and define
, where 1 < ρ < 2, and define
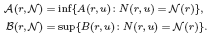
A sharp upper bound is obtained for  and a sharp lower bound is obtained for
and a sharp lower bound is obtained for  .
.
Keywords
MSC classification
Information
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 54 , Issue 3 , October 2011 , pp. 685 - 693
- Copyright
- Copyright © Edinburgh Mathematical Society 2011
References
1.Fenton, P. C. and Rossi, J., Phragmén–Lindelöf theorems, Proc. Am. Math. Soc. 132 (2003), 761–768.CrossRefGoogle Scholar
2.Fenton, P. C. and Rossi, J., cos πρ theorems for δ-subharmonic functions, J. Analyse Math. 92 (2004), 385–396.CrossRefGoogle Scholar

