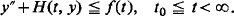Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Wong, James S. W.
1975.
On the Generalized Emden–Fowler Equation.
SIAM Review,
Vol. 17,
Issue. 2,
p.
339.
Kartsatos, Athanassios G.
1976.
On the oscillation problem of nonlinear equations.
Hiroshima Mathematical Journal,
Vol. 6,
Issue. 2,
Kartsatos, Athanassios G.
1976.
Oscillation and existence of unique positive solutions for nonlinear $n$th order equations with forcing term.
Hiroshima Mathematical Journal,
Vol. 6,
Issue. 1,
Kusano, Takaŝi
and
Onose, Hiroshi
1977.
Asymptotic behavior of nonoscillatory solutions of nonlinear differential equations with forcing term.
Annali di Matematica Pura ed Applicata,
Vol. 112,
Issue. 1,
p.
231.
Kartsatos, Athanassios G.
1977.
Analysis of the effect of certain forcings on the nonoscillatory solutions of even order equations.
Journal of the Australian Mathematical Society,
Vol. 24,
Issue. 2,
p.
234.
Staikos, V. A.
1980.
Asymptotic behavior and oscillatory character of bounded solutions of differential equations with deviating arguments.
Ukrainian Mathematical Journal,
Vol. 31,
Issue. 6,
p.
544.
Kusano, Takaŝi
and
Naito, Manabu
1982.
Oscillation Criteria for a Class of Perturbed Schrödinger Equations.
Canadian Mathematical Bulletin,
Vol. 25,
Issue. 1,
p.
71.
Wong, James S. W.
1988.
Second Order Nonlinear Forced Oscillations.
SIAM Journal on Mathematical Analysis,
Vol. 19,
Issue. 3,
p.
667.
Mitrinović, D. S.
Pečarić, J. E.
and
Fink, A. M.
1991.
Inequalities Involving Functions and Their Integrals and Derivatives.
p.
314.
Zafer, A.
and
Dahiya, R. S.
1996.
World Congress of Nonlinear Analysts '92.
p.
1977.
Agarwal, Ravi P.
Grace, Said R.
and
O’Regan, Donal
2002.
Oscillation Theory for Second Order Linear, Half-Linear, Superlinear and Sublinear Dynamic Equations.
p.
625.
Candan, T.
and
Dahiya, R.S.
2004.
Oscillation behavior of nth order neutral differential equations with continuous delay.
Journal of Mathematical Analysis and Applications,
Vol. 290,
Issue. 1,
p.
105.
Candan, T.
and
Dahiya, R.S.
2005.
Oscillation behavior of higher order neutral differential equations.
Applied Mathematics and Computation,
Vol. 167,
Issue. 2,
p.
1267.